Everymaths 15 A Short Introduction To Laplace Transform Pairs And Properties

Everymaths 15 A Short Introduction To Laplace Transform Pairs And Everymaths briefly introduces the laplace transform, the laplace transform pairs, and the properties of laplace transform. We use t as the independent variable for f because in applications the laplace transform is usually applied to functions of time. the laplace transform can be viewed as an operator l that transforms the function f = f(t) into the function f = f(s). thus, equation 7.1.2 can be expressed as. f = l(f).

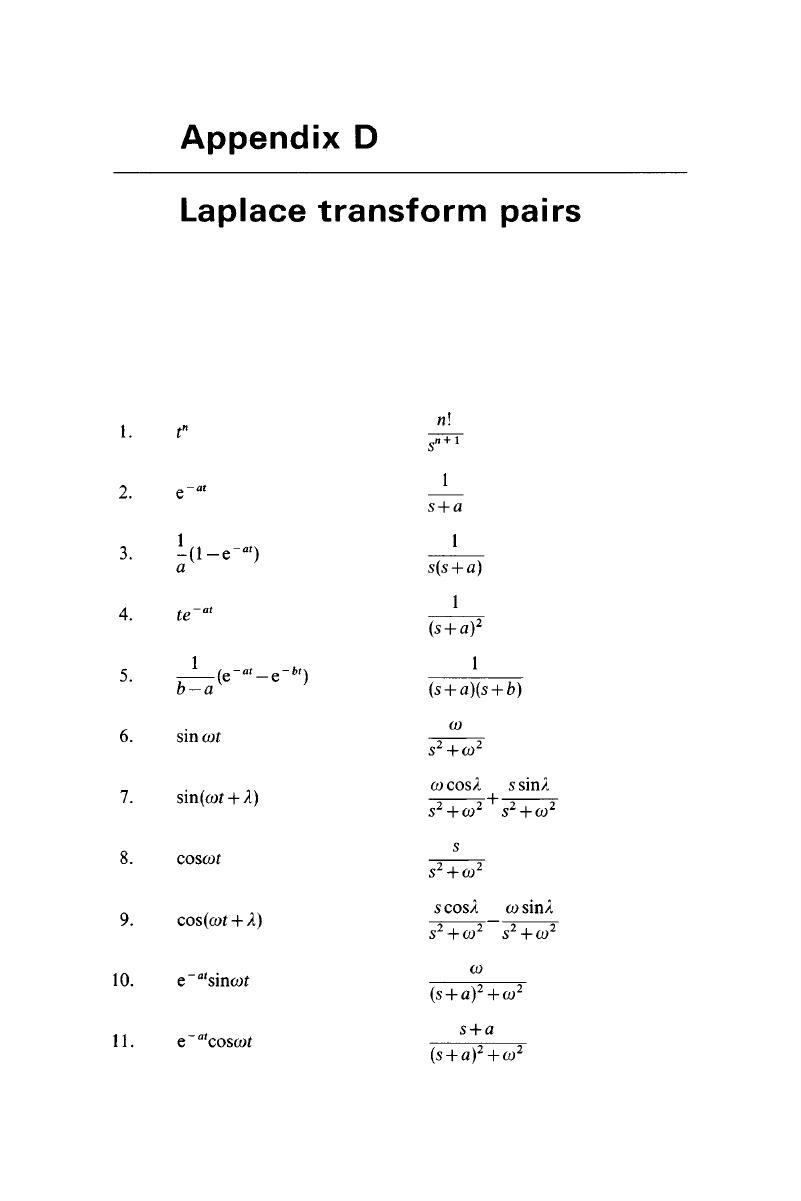
Laplace Transform Pairs D Electrical Circuits Laplace transform is the integral transform of the given derivative function with real variable t to convert into a complex function with variable s. visit byju’s to learn the definition, properties, inverse laplace transforms and examples. It is typical that one makes use of laplace transforms by referring to a table of transform pairs. a sample of such pairs is given in table \(\pageindex{1}\). combining some of these simple laplace transforms with the properties of the laplace transform, as shown in table \(\pageindex{2}\), we can deal with many applications of the laplace. This page titled 13.4: properties of laplace transform is shared under a cc by nc sa 4.0 license and was authored, remixed, and or curated by jeremy orloff (mit opencourseware) via source content that was edited to the style and standards of the libretexts platform. Chapter 4 laplace transforms notes proofread by yunting gao and corrections made on 03 30 2021 4 introduction 4.1 definition and the laplace transform of simple functions given f, a function of time, with value f(t) at time t, the laplace transform of fwhich is denoted by l(f) (or f) is defined by l(f)(s) = f(s) = z 1 0 e stf(t)dt s>0: (1.

Laplace Transform Pairs And Properties Youtube This page titled 13.4: properties of laplace transform is shared under a cc by nc sa 4.0 license and was authored, remixed, and or curated by jeremy orloff (mit opencourseware) via source content that was edited to the style and standards of the libretexts platform. Chapter 4 laplace transforms notes proofread by yunting gao and corrections made on 03 30 2021 4 introduction 4.1 definition and the laplace transform of simple functions given f, a function of time, with value f(t) at time t, the laplace transform of fwhich is denoted by l(f) (or f) is defined by l(f)(s) = f(s) = z 1 0 e stf(t)dt s>0: (1. 2 laplace transform the <0 intuition: the laplace transform is ‘one sided’.1 it sees only the function f(t) in the range t>0:you can think of las acting on functions f(t) ‘set to zero’ for t<0, e.g. l[et] = transform of (0 t<0 et t>0: derivation: the inverse transform requires some explanation due to the contour (later). Expansion, properties of the laplace transform to be derived in this section and summarized in table 4.1, and the table of common laplace transform pairs, table 4.2. the use of the partial fraction expansion method is sufficient for the purpose of this course. however, in general, in order to find the laplace transform of any.