Evaluating Integrals Examples Part 1 Using Infinite Rectangles

Evaluating Integrals Examples Part 1 Using Infinite Rectangles In this video i go through an example in determine the area under a curve using riemann summations and integral notation, which i introduced in my earlier vi. Sr(n) = ∑n i = 1f(xi 1)Δx, the sum of equally spaced rectangles formed using the right hand rule, and. sm(n) = ∑n i = 1f(xi xi 1 2)Δx, the sum of equally spaced rectangles formed using the midpoint rule. recall the definition of a limit as n → ∞: limn → ∞sl(n) = k if, given any ϵ> 0, there exists n> 0 such that.

Calculus Steps In Evaluating Infinite Integral Mathematics Stack 5.2.1 state the definition of the definite integral. 5.2.2 explain the terms integrand, limits of integration, and variable of integration. 5.2.3 explain when a function is integrable. 5.2.4 describe the relationship between the definite integral and net area. 5.2.5 use geometry and the properties of definite integrals to evaluate them. Definite integral. a primary operation of calculus; the area between the curve and the x axis over a given interval is a definite integral. integrable function. a function is integrable if the limit defining the integral exists; in other words, if the limit of the riemann sums as n goes to infinity exists. integrand. Riemann sums help us approximate definite integrals, but they also help us formally define definite integrals. learn how this is achieved and how we can move between the representation of area as a definite integral and as a riemann sum. Definition: definite integral. if f(x) is a function defined on an interval [a, b], the definite integral of f from a to b is given by. ∫b af(x)dx = lim n → ∞ n ∑ i = 1f(x ∗ i)Δx, provided the limit exists. if this limit exists, the function f(x) is said to be integrable on [a, b], or is an integrable function.

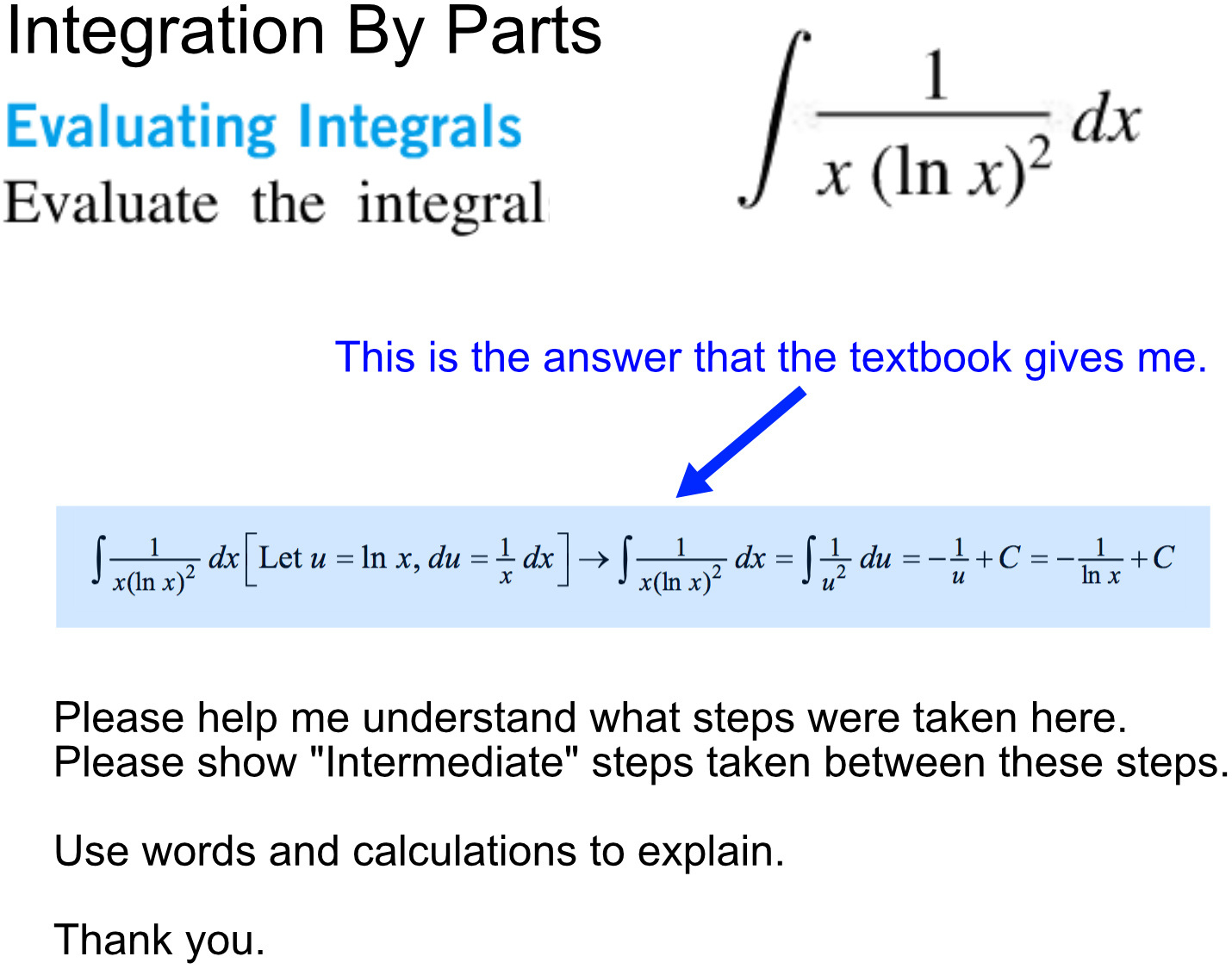
Solved Integration By Parts Evaluating Integrals Evaluate Chegg Riemann sums help us approximate definite integrals, but they also help us formally define definite integrals. learn how this is achieved and how we can move between the representation of area as a definite integral and as a riemann sum. Definition: definite integral. if f(x) is a function defined on an interval [a, b], the definite integral of f from a to b is given by. ∫b af(x)dx = lim n → ∞ n ∑ i = 1f(x ∗ i)Δx, provided the limit exists. if this limit exists, the function f(x) is said to be integrable on [a, b], or is an integrable function. Nd e. timating with finite sums—examples and proofs september. 8, 2020calculu. 1chapter 5. integrals5.1. area and estimating with finite sums—examples and proofsexercise 5.1.6. use rectangles each of whose height is given by the value of the function at the midpoint of the rectangle’s base (the midpoint rule), estimate the area under. It is straightforward to see that any function that is piecewise continuous on an interval of interest will also have a well defined definite integral. definition 4.3.1. the definite integral of a continuous function f on the interval [a, b], denoted ∫b af(x)dx, is the real number given by. ∫b af(x)dx = lim n → ∞ n ∑ i = 1f(x ∗ i.