Euclids Elements Common Notions

Euclid S Elements Common Notions Youtube These common notions, sometimes called axioms, refer to magnitudes of one kind. the various kinds of magnitudes that occur in the elements include lines, angles, plane figures, and solid figures. the first common notion could be applied to plane figures to say, for instance, that if a triangle equals a rectangle, and the rectangle equals a. Common notions. common notion 1. things which equal the same thing also equal one another. common notion 2. if equals are added to equals, then the wholes are equal. common notion 3. if equals are subtracted from equals, then the remainders are equal. common notion 4. things which coincide with one another equal one another. common notion 5.
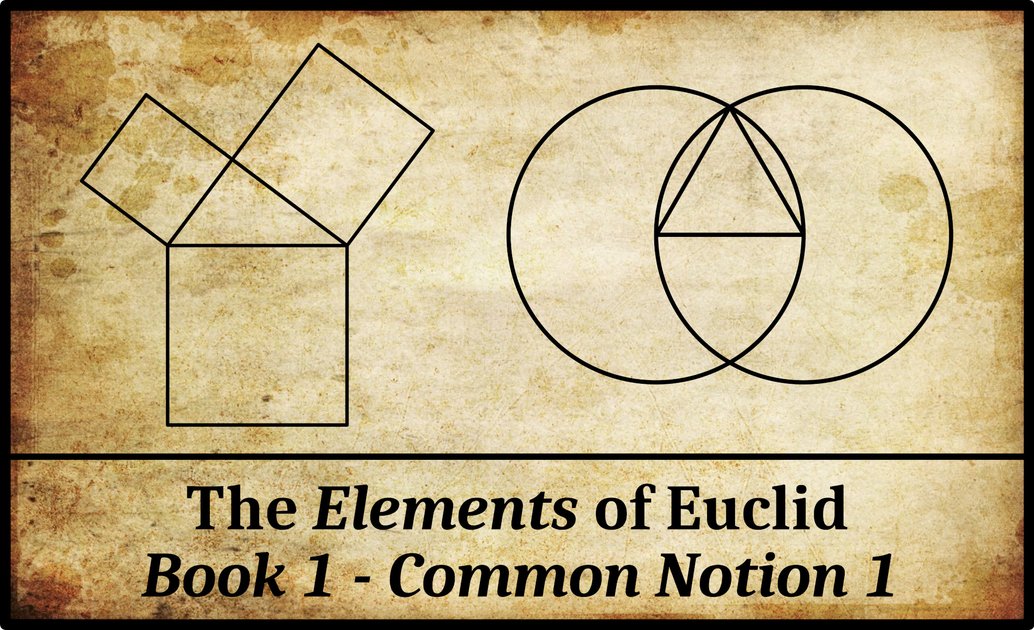
Euclid S Common Notion 1 Peakd The to take is that there is a lot of material in the book, and it’s not especially easy. book i contains 23 definitions, five postulates, five common notions, and 47 propositions. each proposition includes a proof based on the definitions, postulates, common notions, and earlier propositions. although one could study book i sequentially. 1. things which are equal to the same thing are also equal to one another. euclid. euclid's elements. sir thomas little heath. new york. dover. 1956. the national science foundation provided support for entering this text. this work is licensed under a creative commons attribution sharealike 3.0 united states license. Common notions. this is a set of axiomatic statements that appear at the start of book $\text{i}$ of euclid's the elements. common notion 1. in the words of euclid: things which are equal to the same thing are also equal to each other. (the elements: book $\text{i}$: common notions: common notion $1$) common notion 2. in the words of euclid:. Euclid's elements ("stoikheîon") is the foundational text of classical, axiomatic, and deductive geometry ("earth measurement"). the elements is composed of thirteen books, each filled with propositions that beautifully unfold a theory of number, shape, proportion, and measurability. the elements was the essential geomtery textbook for nearly.

Euclid S Axioms And Common Notions Common notions. this is a set of axiomatic statements that appear at the start of book $\text{i}$ of euclid's the elements. common notion 1. in the words of euclid: things which are equal to the same thing are also equal to each other. (the elements: book $\text{i}$: common notions: common notion $1$) common notion 2. in the words of euclid:. Euclid's elements ("stoikheîon") is the foundational text of classical, axiomatic, and deductive geometry ("earth measurement"). the elements is composed of thirteen books, each filled with propositions that beautifully unfold a theory of number, shape, proportion, and measurability. the elements was the essential geomtery textbook for nearly. Euclid's common notions 6 things equal to the same thing are equal. 7 if equals are added to equals, the wholes are equal. 8 if equals are subtracted from equals, the remainders are equal. 9 things that coincide with one another are equal. 10 the whole is greater than a part. Proclus (p. 357, 9) explains that euclid uses the word alternate (or, more exactly, alternately, ἐναλλάξ) in two connexions, (1) of a certain transformation of a proportion, as in book v. and the arithmetical books, (2) as here, of certain of the angles formed by parallels with a straight line crossing them.

Ppt Ma 341 Topics In Geometry Powerpoint Presentation Id 1617227 Euclid's common notions 6 things equal to the same thing are equal. 7 if equals are added to equals, the wholes are equal. 8 if equals are subtracted from equals, the remainders are equal. 9 things that coincide with one another are equal. 10 the whole is greater than a part. Proclus (p. 357, 9) explains that euclid uses the word alternate (or, more exactly, alternately, ἐναλλάξ) in two connexions, (1) of a certain transformation of a proportion, as in book v. and the arithmetical books, (2) as here, of certain of the angles formed by parallels with a straight line crossing them.

Euclid S Elements Common Notions 1 5 Youtube

Comments are closed.