Easiest Proof Of Pythagoras Theorem

How To Prove The Pythagorean Theorem 10 Steps With Pictures What is the pythagorean theorem? you can learn all about the pythagorean theorem, but here is a quick summary: the pythagorean theorem says that, in a right triangle, the square of a (which is a×a, and is written a 2) plus the square of b (b 2) is equal to the square of c (c 2): a 2 b 2 = c 2. proof of the pythagorean theorem using algebra. Pythagoras's proof. given any right triangle with legs a a and b b and hypotenuse c c like the above, use four of them to make a square with sides a b a b as shown below: this forms a square in the center with side length c c and thus an area of c^2. c2. however, if we rearrange the four triangles as follows, we can see two squares inside the.

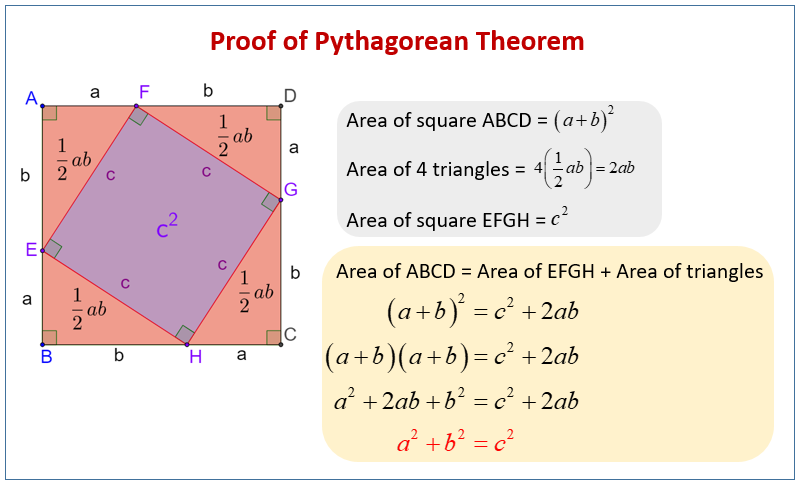
How To Do Garfield S Proof Of The Pythagorean Theorem 10 Steps Some popular dissection proofs of the pythagorean theorem such as proof #36 on cut the knot demonstrate a specific, clear pattern for cutting up the figure's three squares, a pattern that applies to all right triangles. i have yet to find a similarly straightforward cutting pattern that would apply to all triangles and show that my same. 5. set the areas of each arrangement equal to each other. the blue area is a2, the red area, b2 and the green area, c2. the red and blue squares must be added together to equal the area of the green square; therefore, blue area red area = green area: a2 b2 = c2. [6] this finishes the proof. Example 3: use the pythagoras theorem to find the hypotenuse of the triangle in which the sides are 8 units and 6 units respectively. solution: using the pythagoras theorem, hypotenuse 2 = base 2 height 2 = 8 2 6 2. this leads to hypotenuse 2 = 64 36 = 100. therefore, hypotenuse = √100 = 10 units. In mathematics, the pythagorean theorem or pythagoras' theorem is a fundamental relation in euclidean geometry between the three sides of a right triangle. it states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides.
Proving The Pythagorean Theorem Math Liberty Example 3: use the pythagoras theorem to find the hypotenuse of the triangle in which the sides are 8 units and 6 units respectively. solution: using the pythagoras theorem, hypotenuse 2 = base 2 height 2 = 8 2 6 2. this leads to hypotenuse 2 = 64 36 = 100. therefore, hypotenuse = √100 = 10 units. In mathematics, the pythagorean theorem or pythagoras' theorem is a fundamental relation in euclidean geometry between the three sides of a right triangle. it states that the area of the square whose side is the hypotenuse (the side opposite the right angle) is equal to the sum of the areas of the squares on the other two sides. What is the pythagorean theorem? the pythagorean theorem states that the square of the longest side of a right triangle (called the hypotenuse) is equal to the sum of the squares of the other two sides. pythagorean theorem formula shown with triangle abc is: a^2 b^2=c^2 . side c is known as the hypotenuse. the hypotenuse is the longest side of. Pythagorean theorem. download wolfram notebook. for a right triangle with legs and and hypotenuse , (1) many different proofs exist for this most fundamental of all geometric theorems. the theorem can also be generalized from a plane triangle to a trirectangular tetrahedron, in which case it is known as de gua's theorem.

Comments are closed.