Differentiation 3 Basic Rules Of Differentiation The Product And

Differentiation 3 Basic Rules Of Differentiation The Product 3.3.2 apply the sum and difference rules to combine derivatives. 3.3.3 use the product rule for finding the derivative of a product of functions. 3.3.4 use the quotient rule for finding the derivative of a quotient of functions. 3.3.5 extend the power rule to functions with negative exponents. 3.3.6 combine the differentiation rules to find the. To better understand the sequence in which the differentiation rules are applied, we use leibniz notation throughout the solution: f′ (x) = d dx(2x5 7) = d dx(2x5) d dx(7) apply the sum rule. = 2 d dx(x5) d dx(7) apply the constant multiple rule. = 2(5x4) 0 apply the power rule and the constant rule. = 10x4 simplify.

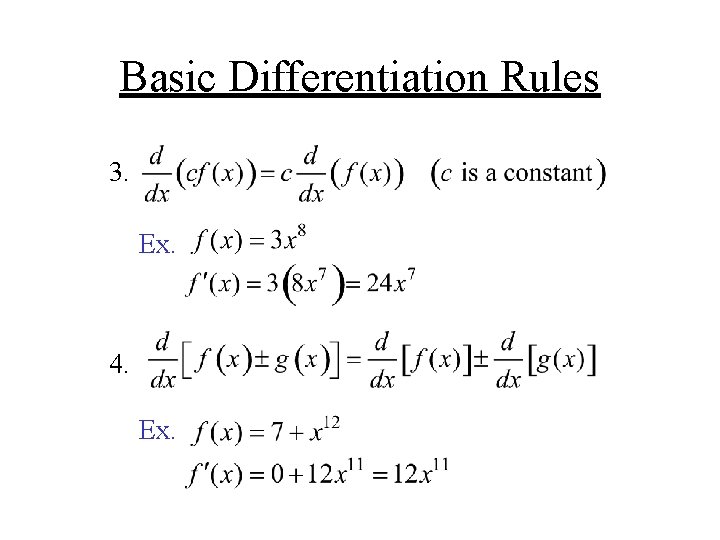
Differentiation 3 Basic Rules Of Differentiation The Product Unit 3 basic differentiation. 3.1 power rule. 3.2 product and quotient rules. 3.3 velocity and other rates of change. 3.4 chain rule. 3.5 trig derivatives. review unit 3. 1.6 determining limits using algebraic manipulation. The first one examines the derivative of the product of two functions. although it might be tempting to assume that the derivative of the product is the product of the derivatives, similar to the sum and difference rules, the product rule does not follow this pattern. to see why we cannot use this pattern, consider the function [latex]f(x)=x^2. Use the product rule to compute the derivative of y = 5x2sinx. evaluate the derivative at x = π 2. solution. to make our use of the product rule explicit, let's set f(x) = 5x2 and g(x) = sinx. we easily compute recall that f′(x) = 10x and g′(x) = cosx. employing the rule, we have d dx(5x2sinx) = 5x2cosx 10xsinx. Apply the sum and difference rules to combine derivatives. compute the derivative of. use the product rule for finding the derivative of a product of functions. use the quotient rule for finding the derivative of a quotient of functions. extend the power rule to functions with negative exponents. combine the differentiation rules to find the.

Differentiation 3 Basic Rules Of Differentiation The Product And Use the product rule to compute the derivative of y = 5x2sinx. evaluate the derivative at x = π 2. solution. to make our use of the product rule explicit, let's set f(x) = 5x2 and g(x) = sinx. we easily compute recall that f′(x) = 10x and g′(x) = cosx. employing the rule, we have d dx(5x2sinx) = 5x2cosx 10xsinx. Apply the sum and difference rules to combine derivatives. compute the derivative of. use the product rule for finding the derivative of a product of functions. use the quotient rule for finding the derivative of a quotient of functions. extend the power rule to functions with negative exponents. combine the differentiation rules to find the. This is called the rule for power functions. if we are given a constant multiple of a function whose derivative we know, or a sum of functions whose derivatives we know, the constant multiple and sum rules make it straightforward to compute the derivative of the overall function. more formally, if f ( x ) and g ( x ) are differentiable with. Differentiation rules are formulae that allow us to find the derivatives of functions quickly. taking derivatives of functions follows several basic rules: multiplication by a constant:.

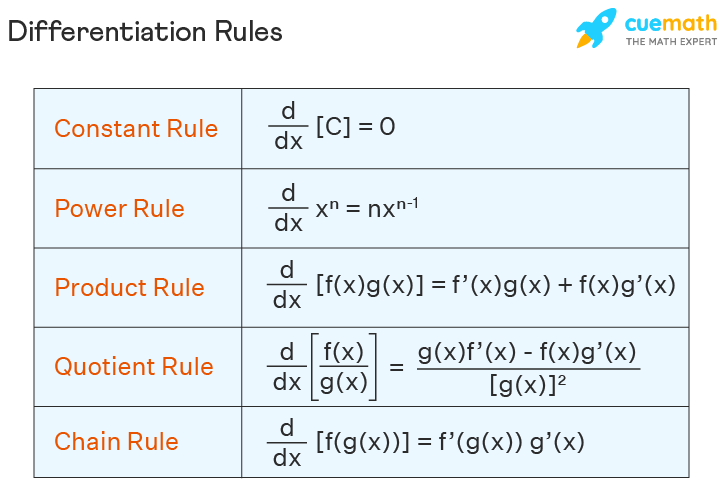
Differentiation Formula Calculus Differentiation Meaning This is called the rule for power functions. if we are given a constant multiple of a function whose derivative we know, or a sum of functions whose derivatives we know, the constant multiple and sum rules make it straightforward to compute the derivative of the overall function. more formally, if f ( x ) and g ( x ) are differentiable with. Differentiation rules are formulae that allow us to find the derivatives of functions quickly. taking derivatives of functions follows several basic rules: multiplication by a constant:.