Differentiating Exponential Functions 1 Of 3 A Visual Argument For

Differentiating Exponential Functions 1 Of 3 A Visual Argument For More resources available at misterwootube. Solution. this problem really makes use of the properties of logarithms and the differentiation rules given in this chapter. \ln y=\ln\dfrac {x\sqrt {2x 1}} {e^x\sin^3 x} step 1. take the natural logarithm of both sides. \ln y=\ln x \frac {1} {2}\ln (2x 1)−x\ln e−3\ln \sin x. step 2.
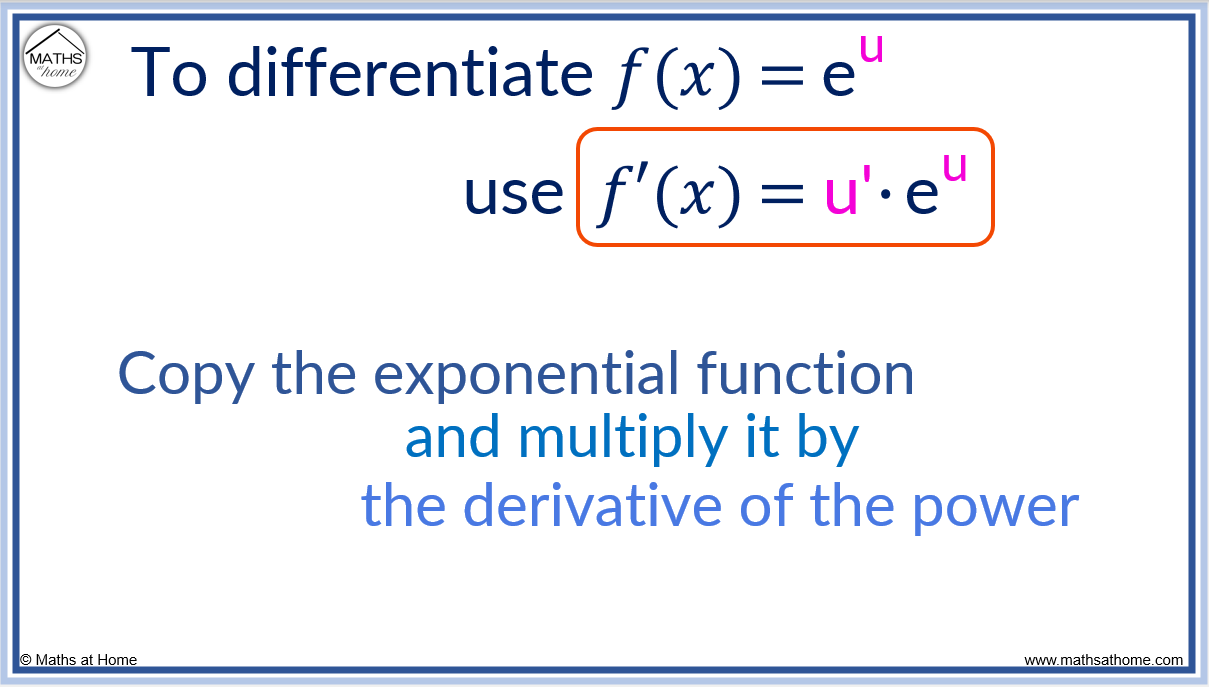
How To Differentiate Exponential Functions Mathsathome Derivatives of exponential functions. in order to differentiate the exponential function. f (x) = a^x, f (x) = ax, we cannot use power rule as we require the exponent to be a fixed number and the base to be a variable. instead, we're going to have to start with the definition of the derivative: \begin {aligned} f' (x) &= \lim {h \rightarrow 0. This page titled 2.7: derivatives of exponential functions is shared under a cc by nc sa 4.0 license and was authored, remixed, and or curated by joel feldman, andrew rechnitzer and elyse yeager via source content that was edited to the style and standards of the libretexts platform. now that we understand how derivatives interact with products. Derivative of the exponential function. just as when we found the derivatives of other functions, we can find the derivatives of exponential and logarithmic functions using formulas. as we develop these formulas, we need to make certain basic assumptions. the proofs that these assumptions hold are beyond the scope of this course. Gcse, a level, pure, mechanics, statistics, discrete – if it’s in a maths exam, paul will know about it. paul is a passionate fan of clear and colourful notes with fascinating diagrams – one of the many reasons he is excited to be a member of the sme team. revision notes on 6.1.4 derivatives of exponential functions for the edexcel a.

How To Differentiate Exponential Functions Mathsathome Derivative of the exponential function. just as when we found the derivatives of other functions, we can find the derivatives of exponential and logarithmic functions using formulas. as we develop these formulas, we need to make certain basic assumptions. the proofs that these assumptions hold are beyond the scope of this course. Gcse, a level, pure, mechanics, statistics, discrete – if it’s in a maths exam, paul will know about it. paul is a passionate fan of clear and colourful notes with fascinating diagrams – one of the many reasons he is excited to be a member of the sme team. revision notes on 6.1.4 derivatives of exponential functions for the edexcel a. A visual estimate of the slopes of the tangent lines to these functions at 0 provides evidence that the value of [latex]e[ latex] lies somewhere between 2.7 and 2.8. the function [latex]e(x)=e^x[ latex] is called the natural exponential function. its inverse, [latex]l(x)=\log e x=\ln x[ latex] is called the natural logarithmic function. A visual estimate of the slopes of the tangent lines to these functions at 0 provides evidence that the value of [latex]e[ latex] lies somewhere between 2.7 and 2.8. the function [latex]e(x)=e^x[ latex] is called the natural exponential function. its inverse, [latex]l(x)=\log e x=\ln x[ latex] is called the natural logarithmic function.

Comments are closed.