Derivative By First Principle Example 2

Derivative By First Principle Example 2 Youtube To do differentiation by first principles: find f(x h) by substituting x with x h in the f(x) equation. substitute f(x h) and f(x) into the first principles equation. simplify the numerator. divide all terms by h. substituting h=0 to evaluate the limit. example: differentiate f(𝑥) = 2𝑥 5 using first principles. we already know that. Calculus fundamentals. understand the mathematics of continuous change. derivative by first principle refers to using algebra to find a general expression for the slope of a curve. it is also known as the delta method. the derivative is a measure of the instantaneous rate of change, which is equal to. \ [ f' (x) = \lim {h \rightarrow 0 } \frac.

How To Find The Derivative Of A X From First Principles Youtube Important: the derivative (also called differentiation) can be written in several ways. this can cause some confusion when we first learn about differentiation. the following are equivalent ways of writing the first derivative of `y = f(x)`: `dy dx` or `f’(x)` or `y’`. example 1. find `dy dx` from first principles if y = 2x 2 3x. answer. After simplifying the function and taking the limit, the derivative of x^3 is found to be 3x^2. first principles example 2: x³ . first principles example 3. First principles of derivatives definition. the first principle of derivatives involves using algebra to determine a general expression for the slope of a curve. it is also referred to as the delta method. the derivative serves as a measure of the instantaneous rate of change, denoted by f'(x), which is equal to:. Step 1: identify the function f (x) and substitute this into the first principles formula. e.g. show, from first principles, that the derivative of 3x2 is 6x. so. step 2: expand f (x h) in the numerator. step 3: simplify the numerator, factorise and cancel h with the denominator.

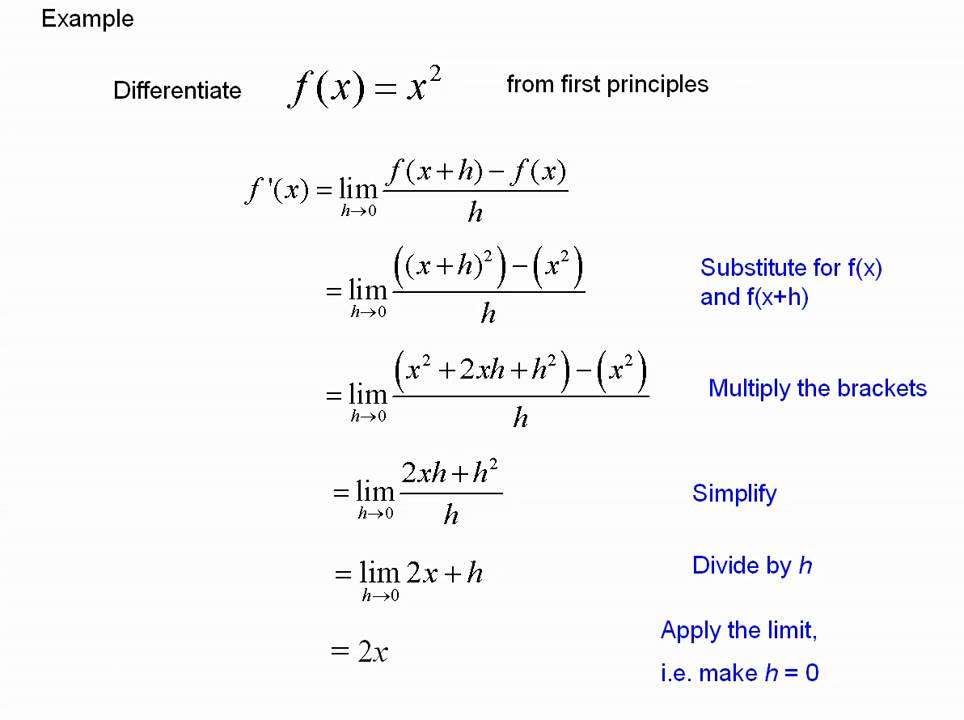
Differentiating From First Principles Youtube First principles of derivatives definition. the first principle of derivatives involves using algebra to determine a general expression for the slope of a curve. it is also referred to as the delta method. the derivative serves as a measure of the instantaneous rate of change, denoted by f'(x), which is equal to:. Step 1: identify the function f (x) and substitute this into the first principles formula. e.g. show, from first principles, that the derivative of 3x2 is 6x. so. step 2: expand f (x h) in the numerator. step 3: simplify the numerator, factorise and cancel h with the denominator. This is the definition, for any function y = f (x), of the derivative, dy dx. note: given y = f (x), its derivative, or rate of change of y with respect to x is defined as. example. suppose we want to differentiate the function f (x) = 1 x from first principles. a sketch of part of this graph shown below. Example 1. if \(f\left(x\right)=x^{2},\) find the derivative of \(f\left(x\right)\) from first principles. solution: using first principles, 1 1 you need to know the identity \[\begin{align*} \left(a b\right)^{2} & =a^{2} 2ab b^{2} \end{align*}\] for this example. in general, you need to know a bit of algebra to do limits effectively.

How To Find The Derivative Of X 2 From First Principles Youtube This is the definition, for any function y = f (x), of the derivative, dy dx. note: given y = f (x), its derivative, or rate of change of y with respect to x is defined as. example. suppose we want to differentiate the function f (x) = 1 x from first principles. a sketch of part of this graph shown below. Example 1. if \(f\left(x\right)=x^{2},\) find the derivative of \(f\left(x\right)\) from first principles. solution: using first principles, 1 1 you need to know the identity \[\begin{align*} \left(a b\right)^{2} & =a^{2} 2ab b^{2} \end{align*}\] for this example. in general, you need to know a bit of algebra to do limits effectively.

4 Derivative By First Principle Example 2 Note Youtube