Corresponding Angles Are Euqal Ll Maths Project

Corresponding Angles Definition Types And Example Cuemath Corresponding angles. corresponding angles are one of the types of angles that are formed when two parallel lines are intersected by the transversal. these are formed in the matching corners or corresponding corners with the transversal. corresponding angles have important applications in the areas of mathematics and physics. 2x = 11. x = 112. x = 5.5. 2. if eg and bd are two parallel lines, find the measure of the angle x. solution: the angles ∠gfh and ∠efc are vertically opposite angles. vertical angles are always congruent. thus, m∠cfe = m∠gfh = 48°. now, the angles ∠efc and ∠bca are corresponding angles formed by parallel lines.
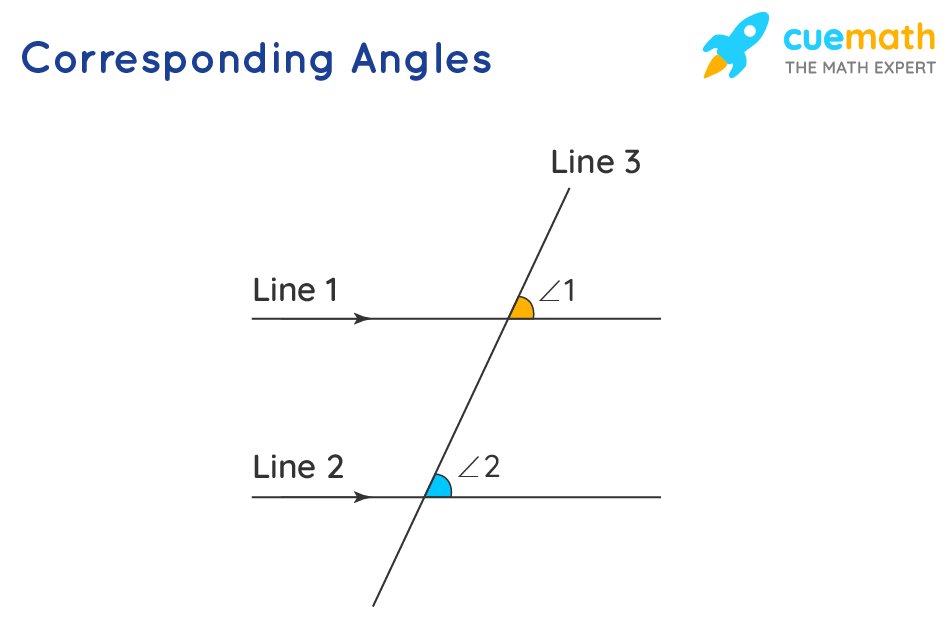
Corresponding Angles Definition Theorem Examples Corresponding angles: suppose that l, m and t are distinct lines. then l and m are parallel if and only if corresponding angles of the intersection of l and t, and m and t are equal. proof: => assume l and m are parallel, prove corresponding angles are equal. assuming l || m, let's label a pair of corresponding angles α and β. Corresponding angles are pairs of angles that occupy the same relative position at each intersection when a transversal intersects two parallel straight lines. corresponding angles. the above figure shows two parallel lines ab and cd intersected by the transversal gh. the pairs of corresponding angles in the given figure are: ∠1 and ∠5. When two lines are crossed by another line (called the transversal): the angles in matching corners are called corresponding angles. in this example a and e are corresponding angles. also: • b and f are corresponding angles. • c and g are corresponding angles. • d and h are corresponding angles. The corresponding angles are opposite angles that share the same measure when two lines intersect. they always have equal measurements and can be used to solve various geometric problems. understanding which angles correspond with each other is important for any student studying geometry as it allows them to simplify their work by only having.
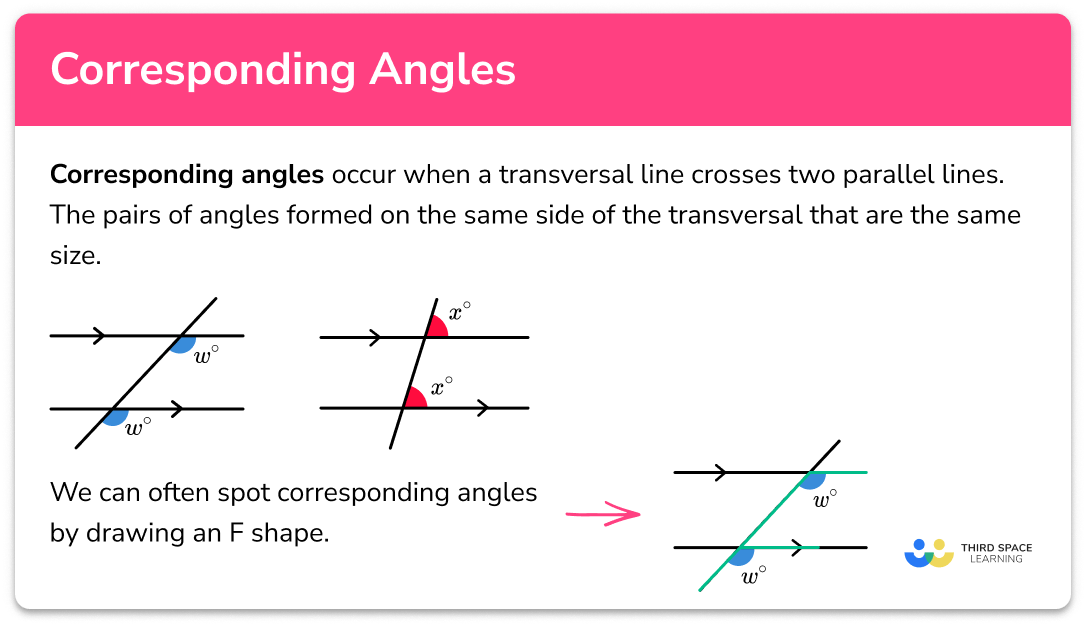
Corresponding Angles Gcse Maths Steps Examples When two lines are crossed by another line (called the transversal): the angles in matching corners are called corresponding angles. in this example a and e are corresponding angles. also: • b and f are corresponding angles. • c and g are corresponding angles. • d and h are corresponding angles. The corresponding angles are opposite angles that share the same measure when two lines intersect. they always have equal measurements and can be used to solve various geometric problems. understanding which angles correspond with each other is important for any student studying geometry as it allows them to simplify their work by only having. Two angles correspond or relate to each other by being on the same side of the transversal. one is an exterior angle (outside the parallel lines), and one is an interior angle (inside the parallel lines). corresponding angles are just one type of angle pair. angles that are on the opposite side of the transversal are called alternate angles. Example 3. the two corresponding angles of a figure measure 7y – 12 and 5y 6. find the magnitude of a corresponding angle. solution. first, we need to determine the value of y. the two corresponding angles are always congruent. hence, 7y – 12 = 5y 6. 7y – 5y = 12 6.
Corresponding Angles Gcse Maths Steps Examples Two angles correspond or relate to each other by being on the same side of the transversal. one is an exterior angle (outside the parallel lines), and one is an interior angle (inside the parallel lines). corresponding angles are just one type of angle pair. angles that are on the opposite side of the transversal are called alternate angles. Example 3. the two corresponding angles of a figure measure 7y – 12 and 5y 6. find the magnitude of a corresponding angle. solution. first, we need to determine the value of y. the two corresponding angles are always congruent. hence, 7y – 12 = 5y 6. 7y – 5y = 12 6.

Comments are closed.