Converting Units With Conversion Factors Metric System Review Dimensional Analysis

Converting Units With Conversion Factors Metric System Review This metric system review video tutorial provides an overview review of how to convert from one unit to another using a technique called dimensional analys. Using the conversion factors given below, calculate the volume of 5.26 l water in gallons. to find the correct conversion factors, first look for a unit that is correlated to liters. in this table, it is the qt which is then linked to gallons. therefore, we can write a two step conversion using dimensional analysis: converting units raised to power.

Converting Units With Conversion Factors Metric System Review In general: the number of units of b = the number of units of a × unit conversion factor. the necessary conversion factors are given in table 1.7.1: 1 lb = 453.59 g; 1 l = 1.0567 qt; 1 l = 1,000 ml. we can convert mass from pounds to grams in one step: 9.26lb × 453.59 g 1lb = 4.20 × 103g. Ratios, sometimes called conversion factors, are fractions that denote the correlation between the given unit and the desired unit. dimensional analysis can be used to solve any conversion problem and allows problems to be easily checked for possible errors. this handout focuses on the most common conversions: metric, chemical, and multi step. In conclusion, understanding how to convert units using conversion factors and utilizing dimensional analysis is crucial for success in mathematics education. the metric system review provided a comprehensive overview of the fundamental units and prefixes used in measurement. 📈 the metric system uses prefixes like kilo (10^3), mega (10^6), and micro (10^ 6), and understanding these is key to converting between metric units. 🔄 when converting units, always start with the given value, identify the correct conversion factors, and then perform the necessary multiplication or division to obtain the result.

Converting Units With Conversion Factors Metric System Review In conclusion, understanding how to convert units using conversion factors and utilizing dimensional analysis is crucial for success in mathematics education. the metric system review provided a comprehensive overview of the fundamental units and prefixes used in measurement. 📈 the metric system uses prefixes like kilo (10^3), mega (10^6), and micro (10^ 6), and understanding these is key to converting between metric units. 🔄 when converting units, always start with the given value, identify the correct conversion factors, and then perform the necessary multiplication or division to obtain the result. Apply a conversion factor to change a value reported in one unit to a corresponding value in a different unit. dimensional analysis uses conversion factors to change the unit in an amount into an equivalent quantity expressed with a different unit. for example, a conversion factor could be used to convert 3.55 meters to centimeters. Use dimensional analysis to solve problems. dimensional analysis (also called factor label method or unit analysis) is used to convert from one set of units to another. this method is used for both simple (feet to inches) and complex (g cm3 to kg gallon) conversions and uses relationships or conversion factors between different sets of units.

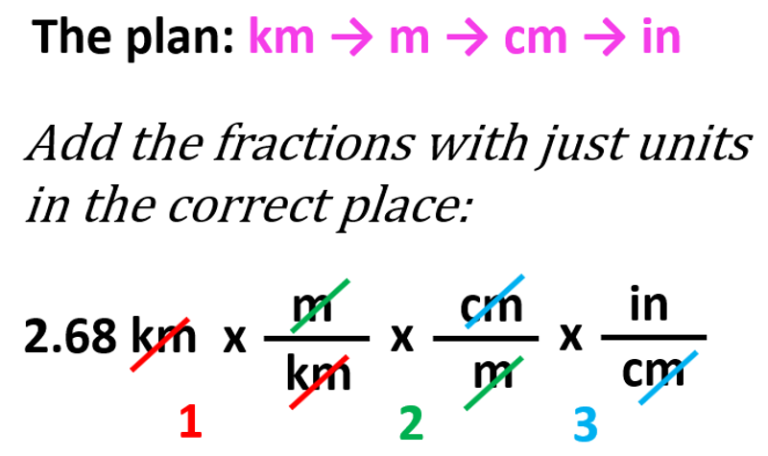
Converting Units With Conversion Factors Dimensional Analysis Apply a conversion factor to change a value reported in one unit to a corresponding value in a different unit. dimensional analysis uses conversion factors to change the unit in an amount into an equivalent quantity expressed with a different unit. for example, a conversion factor could be used to convert 3.55 meters to centimeters. Use dimensional analysis to solve problems. dimensional analysis (also called factor label method or unit analysis) is used to convert from one set of units to another. this method is used for both simple (feet to inches) and complex (g cm3 to kg gallon) conversions and uses relationships or conversion factors between different sets of units.

Learn Metric System Unit Conversions Dimensional Analysis Math

Conversions Dimensional Analysis Notes Youtube