Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240

By gudangdomain On Nov 7, 2024 Last updated

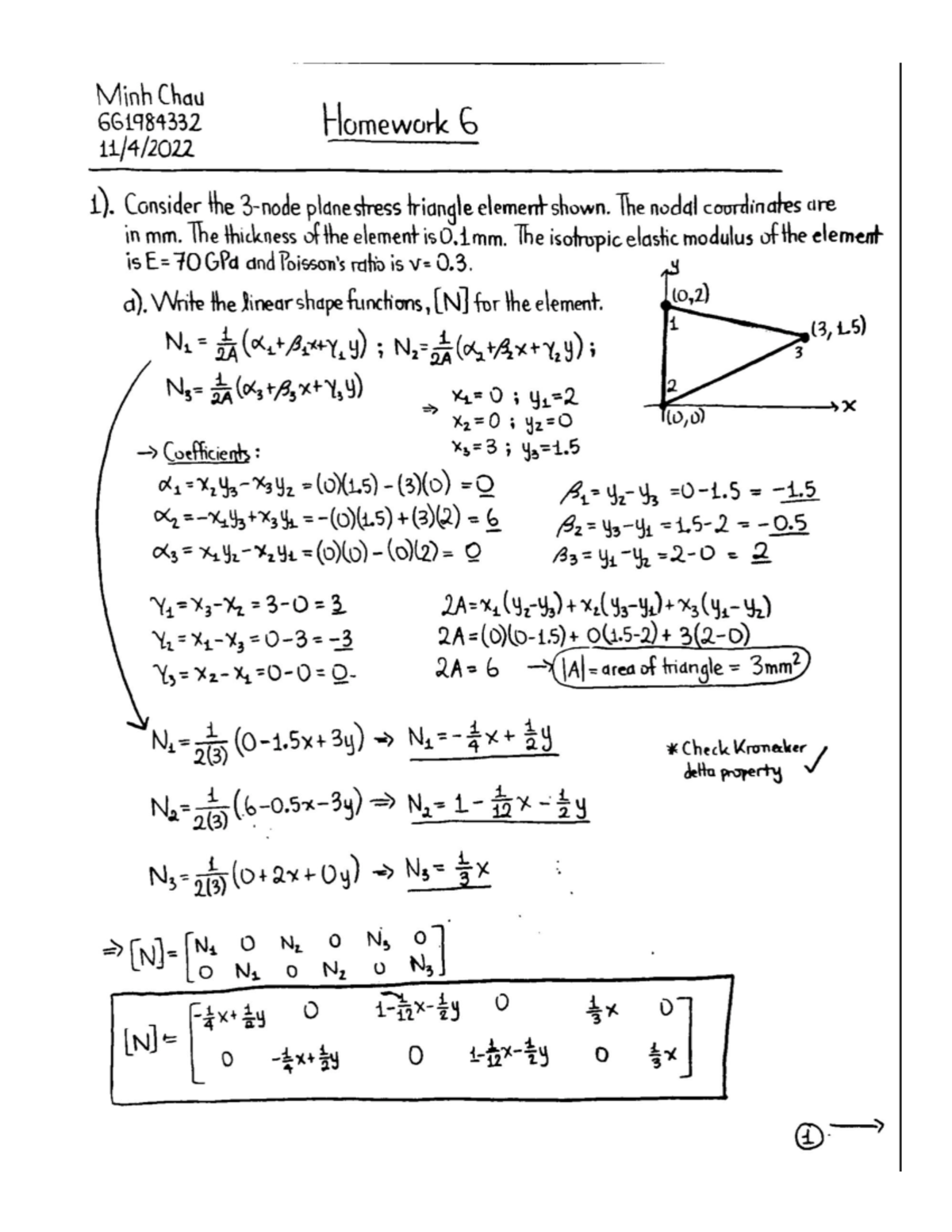
Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240 Exam 1 back exams. activity #3 mat sci. activity #6. activity #5 mat sci minh chau. activity #1. homework 04 answers. activity #4 chaum. solved homework problems on the topic of 2d finite elements, specifically on constant strain triangle (cst) and 2d isoparametric formulation. it includes linear. Microsoft word ch6.doc. me 478 finite element method. chapter 6. isoparametric formulation. same function that is used to define the element geometry is used to define the displacements within the element. 2 node truss element linear geometry linear displacements. 3 node beam element quadratic geometry quadratic displacements.

Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240 Mane 4240: introduction to finite elements (fall 2020) course policy, syllabus and schedule 6 nov fri 5 nov thu 13 fe for 2d elasticity, constant strain triangle 6.1 6.5, ln hw 6 10 nov tue 9 nov mon p5 practicum #5: contact & assembly 13 nov fri 12 nov thu 14 iso parametric formulation (2d), 4 noded quadrilateral 10.2, ln practicum 5 17 nov. View 2d fe cst.pdf from civl 4240 at rensselaer polytechnic institute. mane 4240 & civl 4240 introduction to finite elements 2 d finite elements constant strain triangle (cst) section. Constant strain triangle and 2d isoparametric formulation; 1d finite element shape functions and formulation; hw 3 work practice on using the principle of minimum potential energy, boundary value; hw 1 work given spring assemblies, these two problems ask you to follow the steps learned; lecture 1 springs analysis. The constant strain triangle of chapter 6. hence, the isoparametric formulation was developed. isoparametric elements introduction the isoparametric method may appear somewhat tedious (and confusing initially), but it will lead to a simple computer program formulation, and it is generally applicable for two.

Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240 Constant strain triangle and 2d isoparametric formulation; 1d finite element shape functions and formulation; hw 3 work practice on using the principle of minimum potential energy, boundary value; hw 1 work given spring assemblies, these two problems ask you to follow the steps learned; lecture 1 springs analysis. The constant strain triangle of chapter 6. hence, the isoparametric formulation was developed. isoparametric elements introduction the isoparametric method may appear somewhat tedious (and confusing initially), but it will lead to a simple computer program formulation, and it is generally applicable for two. Mane 4240 & civl 4240 introduction to finite elements. mane 4240 & civl 4240 introduction to finite elements. prof. suvranu de. constant strain triangle (cst). reading assignment: logan 6.2 6.5 lecture notes. summary: computation of shape functions for constant strain triangle properties of the shape functions. 495 views • 33 slides. This chapter explains how to derive 2d solid elements of arbitrary shape (i.e. irregular quadrilaterals and curve sided triangles) using an isoparametric formulation and numerical integration. the basis of the isoparametric formulation for 2d solid elements is described in the next section. then, the quadrature rules for the numerical.

Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240 Mane 4240 & civl 4240 introduction to finite elements. mane 4240 & civl 4240 introduction to finite elements. prof. suvranu de. constant strain triangle (cst). reading assignment: logan 6.2 6.5 lecture notes. summary: computation of shape functions for constant strain triangle properties of the shape functions. 495 views • 33 slides. This chapter explains how to derive 2d solid elements of arbitrary shape (i.e. irregular quadrilaterals and curve sided triangles) using an isoparametric formulation and numerical integration. the basis of the isoparametric formulation for 2d solid elements is described in the next section. then, the quadrature rules for the numerical.

Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240

Embark on a thrilling expedition through the wonders of science and marvel at the infinite possibilities of the universe. From mind-boggling discoveries to mind-expanding theories, join us as we unlock the mysteries of the cosmos and unravel the tapestry of scientific knowledge in our Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240 section.

Finite Element Method: Lecture 17C - 2D Isoparametric Second Order Triangular Element

Finite Element Method: Lecture 17C - 2D Isoparametric Second Order Triangular Element Finite Element Method: Lecture 17B - 2D Isoparametric Linear Triangular Element ENGR 570 Lecture 13: Isoparametric Formulations (2016.02.25) 2-D Elements Finite Element Methods - Constant Strain Triangle (Part 1) Finite Element Method: Lecture 17A - 2D Isoparametric Quadrilateral Bilinear Element 2D Analysis - 4 Noded Quadrilateral element Isoparametric Quadratic elements in Finite Element Method | FEA Problems Finite Element Method Lec 11 - Part 2 - Isoparametric Mapping 3-node Constant Strain Triangle Finite Element-2D CST part 3 Stress Analysis using Constant Strain Triangle CST - Finite Element Analysis Isoparametric triangular elements in FEM | Analysis of Higher order elements | 🔥🔥🔥 FEA-Unit-4-Lecture-7 2:4 1D Isoparametric Elements Intro to the Finite Element Method Lecture 5 | Triangular and Quadrilateral Continuum Elements 2:3 2D Triangular Constant Gradient Elements 77 Second Webinars: Flex Core Separation Distance 2:5 Fluid Flow (H) - Numerical integration, 1D and 2D isoparametric elements

Conclusion

All things considered, it is unmistakable that this specific content delivers beneficial details about Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240. All the way through, the creator displays remarkable understanding in the field. Distinctly, the explanation about this detail stands out as especially noteworthy. In addition, the text is remarkable in clarifying complex concepts in an comprehensible manner. In addition, the blogger includes practical examples that amplify the engagement. Another facet that differentiates this write-up is the rigorous inspection of an array of aspects related to Constant Strain Triangle And 2d Isoparametric Formulation Mane 4240. The scribes meticulous approach warrants that readership get a well-balanced knowledge of the subject matter. Thanks for considering this article. For more details, you are welcome to contact me through comments or email. I am enthusiastic about your comments. In wrapping up, to delve deeper, you will find a number of connected articles that are potentially engaging:Hope you find them interesting!