Classifying A Polynomial

Algebra 2 Classify Polynomials Youtube This wikihow guide shows you how to classify polynomials. things you should know. a term is a coefficient, variable, or both. monomials have 1 term. binomials have 2 terms. trinomials have 3 terms. classify by degree by identifying the highest exponent in the polynomial. method 1. classify by terms. Polynomials can be classified by the degree of the polynomial. the degree of a polynomial is the degree of its highest degree term. so the degree of 2x3 3x2 8x 5 2 x 3 3 x 2 8 x 5 is 3. a polynomial is said to be written in standard form when the terms are arranged from the highest degree to the lowest degree.

8 1 Classifying Polynomials Youtube To classify the polynomial equations, i always start by observing the terms. a term consists of a coefficient and a variable raised to a power, which is referred to as its degree. a polynomial might have one or multiple terms, each made distinct by their coefficients or exponents. for instance, in the polynomial 7 x 2 3 x 2, there are three. The standard form for writing a polynomial is to put the terms with the highest degree first. example: put this in standard form: 3 x2 − 7 4 x3 x6. the highest degree is 6, so that goes first, then 3, 2 and then the constant last: x6 4 x3 3 x2 − 7. you don't have to use standard form, but it helps. A polynomial is a special algebraic expression with terms that consist of real number coefficients and variable factors with whole number exponents. examplesofpolynomials: 3x2 7xy 5 3 2x3 3x2 − 1 2x 1 6x2y − 4xy3 − 4xy3 7. polynomials do not have variables in the denominator of any term. examplesthatarenotpolynomials:. Based on the degree of a polynomial, it can be classified into 4 types: zero polynomial, linear polynomial, quadratic polynomial, cubic polynomial. polynomials should have a whole number as the degree. expressions with negative exponents are not polynomials. for example, x 2 is not a polynomial.
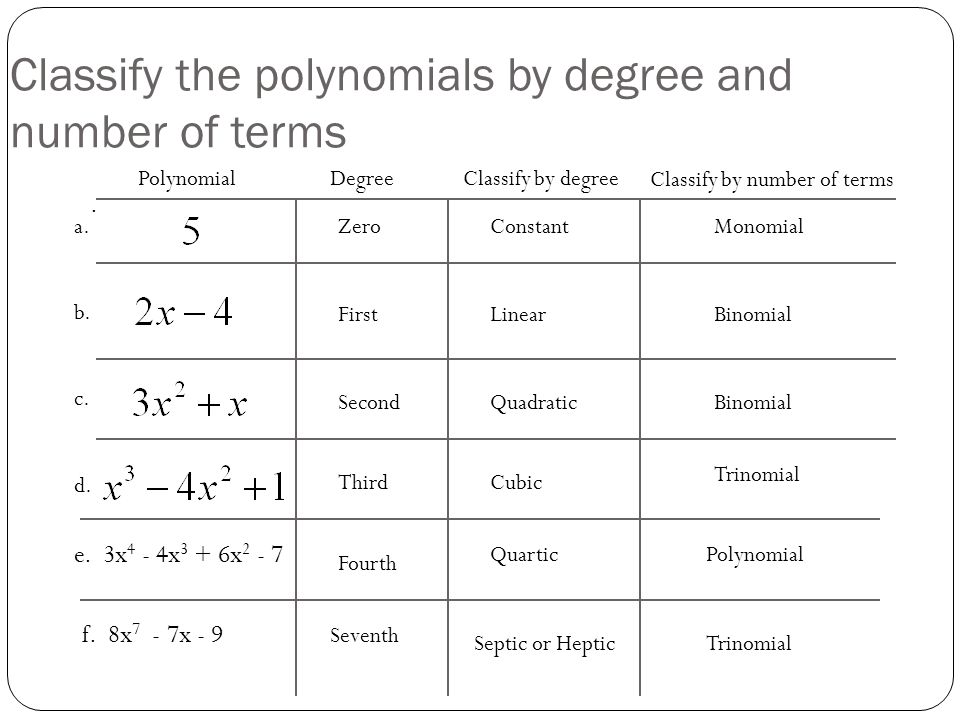
Classifying Polynomials A polynomial is a special algebraic expression with terms that consist of real number coefficients and variable factors with whole number exponents. examplesofpolynomials: 3x2 7xy 5 3 2x3 3x2 − 1 2x 1 6x2y − 4xy3 − 4xy3 7. polynomials do not have variables in the denominator of any term. examplesthatarenotpolynomials:. Based on the degree of a polynomial, it can be classified into 4 types: zero polynomial, linear polynomial, quadratic polynomial, cubic polynomial. polynomials should have a whole number as the degree. expressions with negative exponents are not polynomials. for example, x 2 is not a polynomial. What is a polynomial? polynomial are sums (and differences) of polynomial "terms". for an expression to be a polynomial term, any variables in the expression must have whole number powers (or else the "understood" power of 1, as in x1, which is normally written as x). a plain number can also be a polynomial term. Table 1.6.1. the degree of a term113 in a polynomial is defined to be the exponent of the variable, or if there is more than one variable in the term, the degree is the sum of their exponents. recall that x0 = 1; any constant term can be written as a product of x0 and itself. hence the degree of a constant term is 0.

Ppt Classifying Polynomials Powerpoint Presentation Free Download What is a polynomial? polynomial are sums (and differences) of polynomial "terms". for an expression to be a polynomial term, any variables in the expression must have whole number powers (or else the "understood" power of 1, as in x1, which is normally written as x). a plain number can also be a polynomial term. Table 1.6.1. the degree of a term113 in a polynomial is defined to be the exponent of the variable, or if there is more than one variable in the term, the degree is the sum of their exponents. recall that x0 = 1; any constant term can be written as a product of x0 and itself. hence the degree of a constant term is 0.

Ppt An Intro To Polynomials Powerpoint Presentation Id 3347938

Comments are closed.