Circles Angle Measures Arcs Central Inscribed Angles Tangents Secants Chords Geometry

Arcs And Angles Of Circles Video In the case of a pentagon, the interior angles have a measure of (5 2) •180 5 = 108 °. therefore, each inscribed angle creates an arc of 216° use the inscribed angle formula and the formula for the angle of a tangent and a secant to arrive at the angles. This geometry video tutorial goes deeper into circles and angle measures. it covers central angles, inscribed angles, arc measure, tangent chord angles, cho.

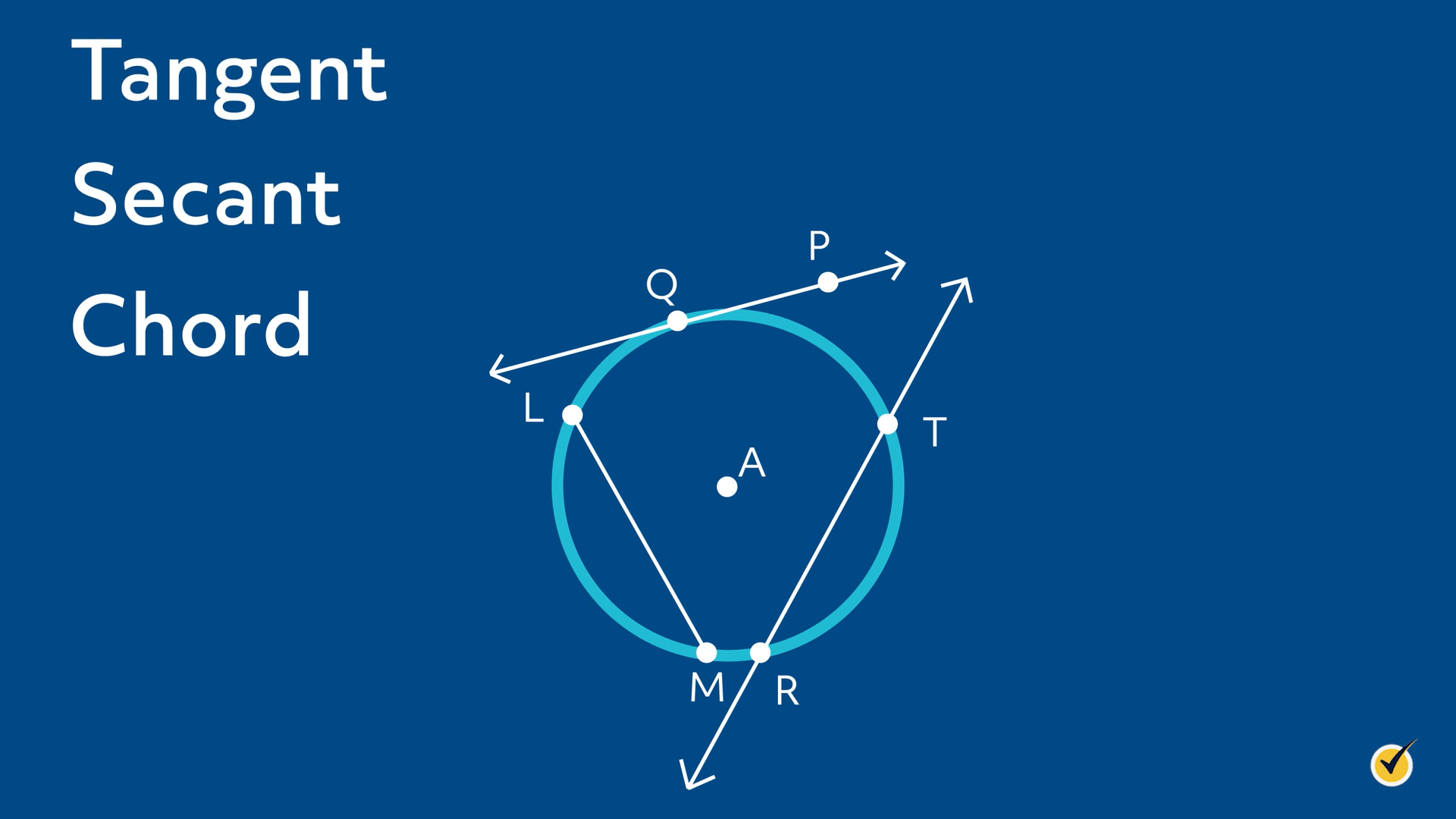
Chords Secants And Tangents Oh My Systry 1. central angle. a central angle is an angle formed by two radii with the vertex at the center of the circle. central angle = intercepted arc. in the diagram at the right, ∠aob is a central angle with an intercepted minor arc from a to b. m∠aob = 82º. in a circle, or congruent circles, congruent central angles have congruent arcs. The formula. the angle formed by the intersection of 2 tangents, 2 secants or 1 tangent and 1 secant outside the circle equals half the difference of the intercepted arcs! therefore to find this angle (angle k in the examples below), all that you have to do is take the far intercepted arc and near the smaller intercepted arc and then divide. Example 1: central angle theorem. find the measure of angle a a in circle d. d. recall the theorem. angle a a is an inscribed angle and is half the measure of the arc it intercepts. 2 solve the problem. arc pc pc is the intercepted arc of angle a. a. arc pc=42^ {\circ} pc = 42∘ and angle a a is an inscribed angle. Thm 10.13: angles outside the circle. if a tangent and a secant, two tangents or two secants intersect outside a circle, then the measure of the angle formed is one half the difference of the measures of the intercepted arcs. in the diagram below, d, e, f and g lie on the circle. thus m ∠ d m ∠ f = 180 ° and.

Circles Angle Measures Arcs Central Inscribed Angles Tangents Example 1: central angle theorem. find the measure of angle a a in circle d. d. recall the theorem. angle a a is an inscribed angle and is half the measure of the arc it intercepts. 2 solve the problem. arc pc pc is the intercepted arc of angle a. a. arc pc=42^ {\circ} pc = 42∘ and angle a a is an inscribed angle. Thm 10.13: angles outside the circle. if a tangent and a secant, two tangents or two secants intersect outside a circle, then the measure of the angle formed is one half the difference of the measures of the intercepted arcs. in the diagram below, d, e, f and g lie on the circle. thus m ∠ d m ∠ f = 180 ° and. A chord is a line segment whose endpoints lie on the circumference of a circle. a tangent is a line that touches a circle at exactly one point. this is called the point of tangency. an arc is a section of the circumference of a circle. a sector is a part of the interior of a circle, bounded by an arc and two radii. Find the degree measure for each angle or arc (problems #20 24) solve for x given intersecting chords, secants or tangents (problems #25 27) find the indicated angle, arc and segment length (problem #28) master circle geometry with our comprehensive guide, covering key concepts, theorems, and step by step examples for success.

Arcs Chords Central Angles And Inscribed Angles Of A Circle Youtube A chord is a line segment whose endpoints lie on the circumference of a circle. a tangent is a line that touches a circle at exactly one point. this is called the point of tangency. an arc is a section of the circumference of a circle. a sector is a part of the interior of a circle, bounded by an arc and two radii. Find the degree measure for each angle or arc (problems #20 24) solve for x given intersecting chords, secants or tangents (problems #25 27) find the indicated angle, arc and segment length (problem #28) master circle geometry with our comprehensive guide, covering key concepts, theorems, and step by step examples for success.

How Are Tangent Secant And Chord Of A Circle Related