Circle Theorems Alternate Segment Theorem Worked Solutions Examples
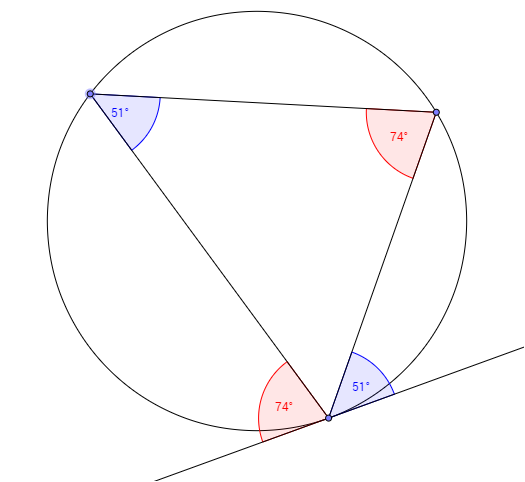
Circle Theorems Alternate Segment Theorem Worked Solutions Examples The alternate segment theorem states. an angle between a tangent and a chord through the point of contact is equal to the angle in the alternate segment. recall that a chord is any straight line drawn across a circle, beginning and ending on the curve of the circle. in the following diagram, the chord ce divides the circle into 2 segments. Go through the following examples to understand the concept of the alternate segment theorem. example 1: find the unknown angles in the figure, given that the chord bc makes the angles 65° with the tangent line pq. solution: given that, ∠qcb = 65°. by using the alternate segment theorem, we can say.
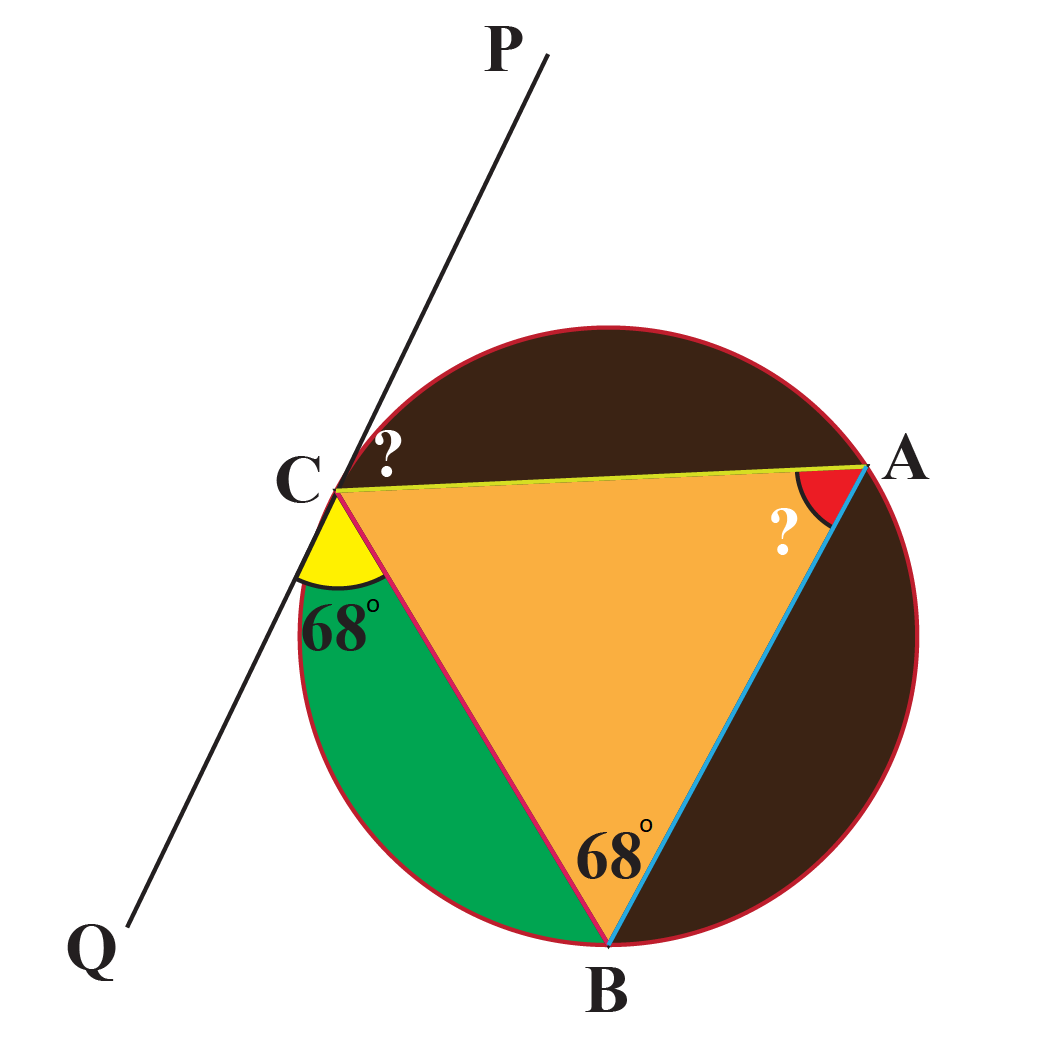
Alternate Segment Theorem Circles Proof Solutions Cuemath Alternate segment theorem. the segment of a circle is the region between a chord and the corresponding arc of the circle. when a chord is drawn, it creates a major segment and a minor segment in the circle. let's observe the figure given below, in which de is the tangent and bc is a chord. ∠ ∠ bce is made by the tangent and chord bc. Alternate segment theorem. here we will learn about the alternate segment theorem, including their application, proof, and using them to solve more difficult problems. there are also circle theorem worksheets based on edexcel, aqa and ocr exam questions, along with further guidance on where to go next if you’re still stuck. Example 1: the alternate segment theorem. the triangle abc is inscribed in a circle with centre o. the tangent de meets the circle at the point a. calculate the size of the angle abc. locate the key parts of the circle for the theorem. here we have: the tangent de. Circle theorems part 1 of 2. the angle between a radius and a tangent is 90 degrees. the angle at the centre is twice the angle at the circumference. angles in the same segment are equal. the angle in a semi circle is always 90 degrees. the opposite angles in a cyclic quadrilateral always add up to 180 degrees.

Alternate Segment Theorem Circles Proof Solutions Cuemath Example 1: the alternate segment theorem. the triangle abc is inscribed in a circle with centre o. the tangent de meets the circle at the point a. calculate the size of the angle abc. locate the key parts of the circle for the theorem. here we have: the tangent de. Circle theorems part 1 of 2. the angle between a radius and a tangent is 90 degrees. the angle at the centre is twice the angle at the circumference. angles in the same segment are equal. the angle in a semi circle is always 90 degrees. the opposite angles in a cyclic quadrilateral always add up to 180 degrees. The alternate segment theorem can only be used for circles. the alternate segment theorem can only be used when there is a chord of the circle, a tangent is passing through one or both of the endpoints of the chord, and the angles that are used in the question are the angle between the chord and the tangent and the angle formed by the chord in. The alternate segment circle theorem is a fundamental theorem in circle geometry. this theorem relates the angles formed by a tangent and a chord at the point of contact with the circle. according to the theorem, the angle between the tangent and the chord at the point of contact is equal to the angle in the alternate segment of the circle.
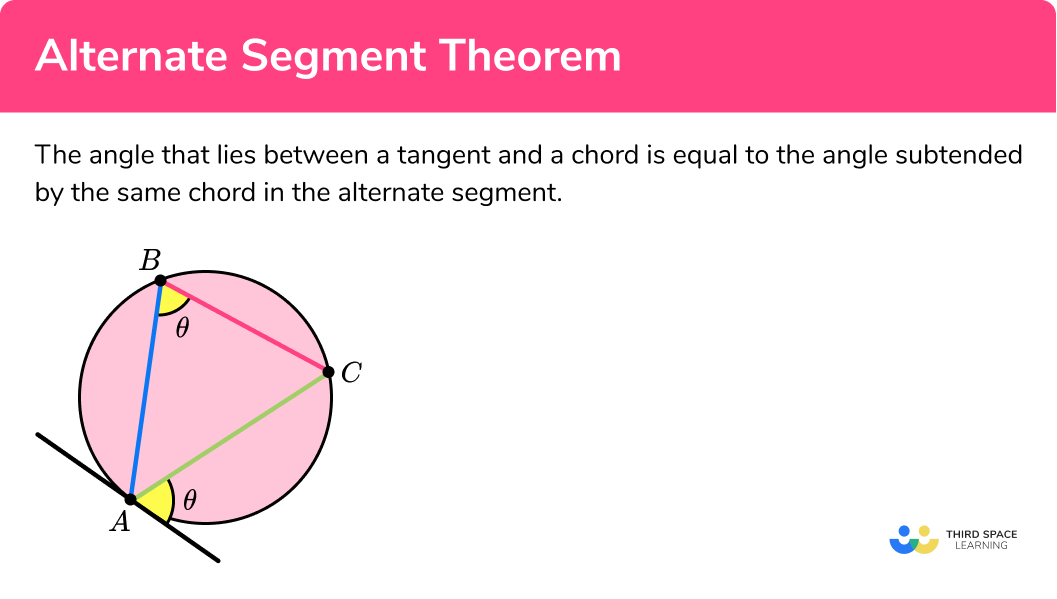
Alternate Segment Theorem Gcse Maths Steps Examples The alternate segment theorem can only be used for circles. the alternate segment theorem can only be used when there is a chord of the circle, a tangent is passing through one or both of the endpoints of the chord, and the angles that are used in the question are the angle between the chord and the tangent and the angle formed by the chord in. The alternate segment circle theorem is a fundamental theorem in circle geometry. this theorem relates the angles formed by a tangent and a chord at the point of contact with the circle. according to the theorem, the angle between the tangent and the chord at the point of contact is equal to the angle in the alternate segment of the circle.

Alternate Segment Theorem Circles Proof Solutions Cuemath

Comments are closed.