Calculus 1 Derivatives

By gudangdomain On Nov 7, 2024 Last updated

Calculus 1 Derivatives Youtube Unit 2 derivatives: definition and basic rules. unit 3 derivatives: chain rule and other advanced topics. unit 4 applications of derivatives. unit 5 analyzing functions. unit 6 integrals. unit 7 differential equations. unit 8 applications of integrals. course challenge. test your knowledge of the skills in this course. Learn how to find and interpret derivatives of various functions using differentiation formulas, rules and methods. explore the applications of derivatives in related rates, implicit differentiation and higher order derivatives.

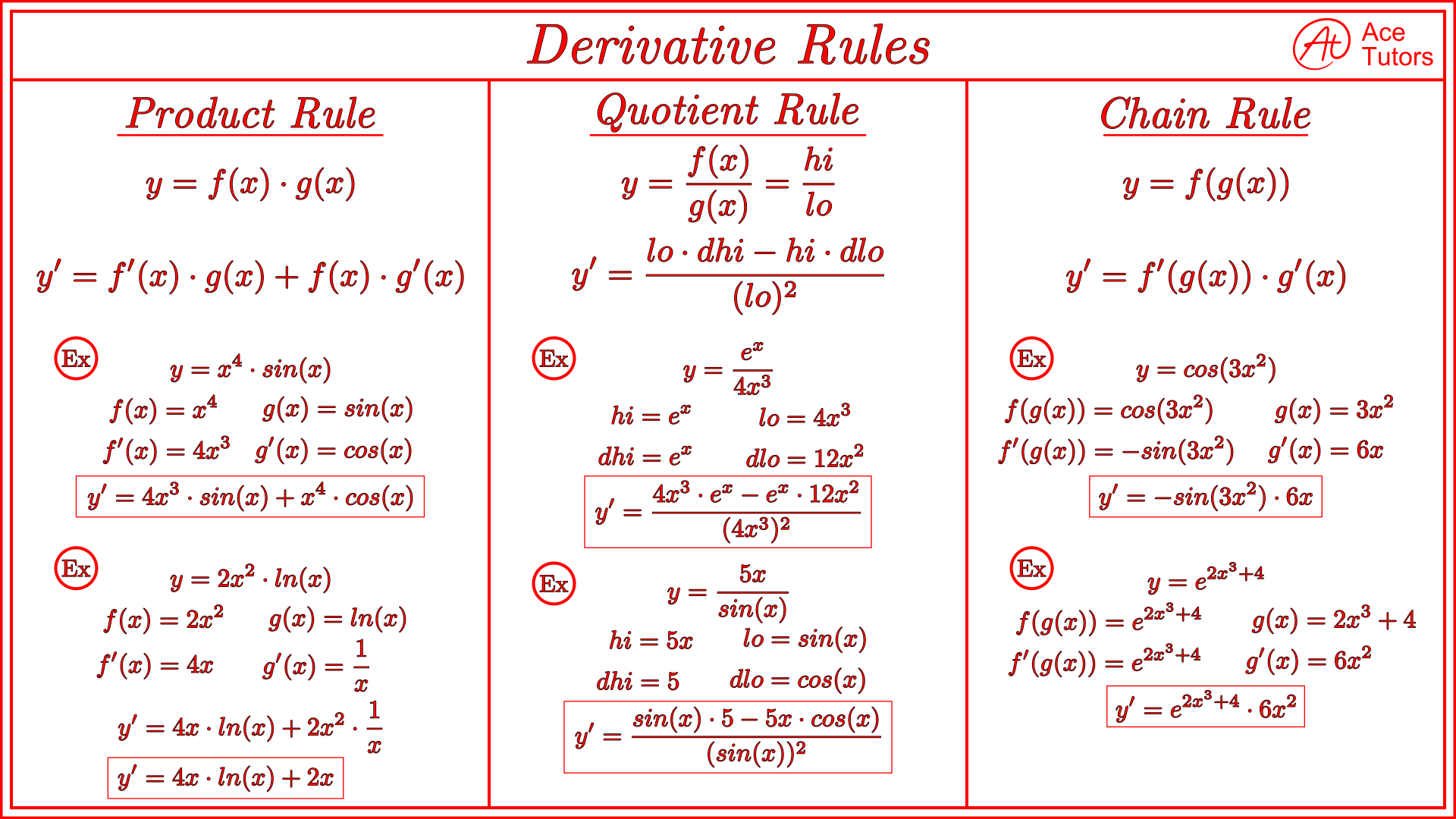
Derivative Rules Cheat Sheet Calculus Ace Tutors Blog Learn the fundamentals of calculus 1 with comprehensive math courses on khan academy. This calculus 1 video tutorial provides a basic introduction into derivatives. full 1 hour 35 minute video: patreon mathsciencetutor. Example: what is the derivative of x 2 x 3 ? the sum rule says: the derivative of f g = f’ g’ so we can work out each derivative separately and then add them. using the power rule: ddx x 2 = 2x; ddx x 3 = 3x 2; and so: the derivative of x 2 x 3 = 2x 3x 2. Here is a set of practice problems to accompany the differentiation formulas section of the derivatives chapter of the notes for paul dawkins calculus i course at lamar university.

Calculus 1 Derivatives Practice Example: what is the derivative of x 2 x 3 ? the sum rule says: the derivative of f g = f’ g’ so we can work out each derivative separately and then add them. using the power rule: ddx x 2 = 2x; ddx x 3 = 3x 2; and so: the derivative of x 2 x 3 = 2x 3x 2. Here is a set of practice problems to accompany the differentiation formulas section of the derivatives chapter of the notes for paul dawkins calculus i course at lamar university. 3.1.3 identify the derivative as the limit of a difference quotient. 3.1.4 calculate the derivative of a given function at a point. 3.1.5 describe the velocity as a rate of change. 3.1.6 explain the difference between average velocity and instantaneous velocity. 3.1.7 estimate the derivative from a table of values. 3.3.1 state the constant, constant multiple, and power rules. 3.3.2 apply the sum and difference rules to combine derivatives. 3.3.3 use the product rule for finding the derivative of a product of functions. 3.3.4 use the quotient rule for finding the derivative of a quotient of functions. 3.3.5 extend the power rule to functions with negative.

We understand that the online world can be overwhelming, with countless sources vying for your attention. That's why we strive to stand out from the crowd by delivering well-researched, high-quality content that not only educates but also entertains. Our articles are designed to be accessible and easy to understand, making complex topics digestible for everyone.

Conclusion

Considering all the aspects, it becomes apparent that the content imparts helpful understanding about Calculus 1 Derivatives. Across the whole article, the essayist illustrates a wealth of knowledge pertaining to the theme. Particularly, the segment on Z stands out as a main highlight. Whats more, the article excels in clarifying complex concepts in an plain manner. On top of that, the creator includes real-world cases that heighten the understanding. Another aspect that makes this piece exceptional is the in-depth research of various aspects related to Calculus 1 Derivatives. The scribes careful style ensures that browsers obtain a comprehensive insight of the subject matter. Thank you for delving into the write-up. Should you require additional details, dont hesitate to make contact leveraging email. I look forward to your comments. In bringing things to a close, if youre interested in further reading, here are a number of connected pieces of content that you will find informative:Hope you enjoy them!