Basic Trigonometric Substitution Shorts

Basic Trigonometric Substitution Shorts Youtube @wallacestem walks us through the basics of trigonometric substitution, including a helpful identity to learn.get more homework help from chegg at ch. Here is a summary for the sine trig substitution. √a2 − b2x2 ⇒ x = a bsinθ, − π 2 ≤ θ ≤ π 2. there is one final case that we need to look at. the next integral will also contain something that we need to make sure we can deal with. example 5 evaluate the following integral. ∫ 1 60 x5 (36x2 1)3 2 dx. show solution.

Trigonometry Substitution Trigonometry Nda Exam Substitution For Example 6.4.6: using trigonometric substitution. evaluate ∫ 1 (x2 6x 10)2 dx. solution. we start by completing the square, then make the substitution u = x 3, followed by the trigonometric substitution of u = tanθ: ∫ 1 (x2 6x 10)2 dx = ∫ 1 ((x 3)2 1)2 dx = ∫ 1 (u2 1)2 du. now make the substitution u = tanθ, du = sec2θ. Welcome to our collection of free calculus lessons and videos. the following diagram shows how to use trigonometric substitution involving sine, cosine, or tangent. scroll down the page for more examples and solutions on the use of trigonometric substitution. trigonometric substitution example 1. just a basic trigonometric substitution problem. Trigonometric substitution common trig substitutions: the following is a summary of when to use each trig substitution. integral contains: substitution domain identity √ a2 −x 2x = asin(θ) −π 2, π 2 1−sin (θ) = cos2 (θ) √ a2 x2 x = atan(θ) − π 2, 2 1 tan2 (θ) = sec2 (θ) √ x2 −a2 x = asec(θ) 0, π 2 sec2 (θ) −1. Figure 7.3.7: calculating the area of the shaded region requires evaluating an integral with a trigonometric substitution. we can see that the area is a = ∫5 3√x2 − 9dx. to evaluate this definite integral, substitute x = 3secθ and dx = 3secθtanθdθ. we must also change the limits of integration.

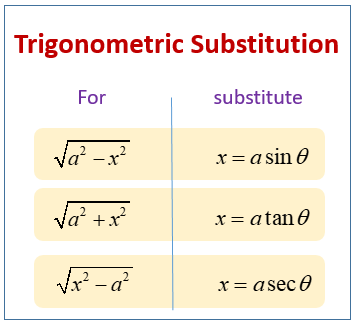
Trigonometric Substitution Examples Solutions Videos Trigonometric substitution common trig substitutions: the following is a summary of when to use each trig substitution. integral contains: substitution domain identity √ a2 −x 2x = asin(θ) −π 2, π 2 1−sin (θ) = cos2 (θ) √ a2 x2 x = atan(θ) − π 2, 2 1 tan2 (θ) = sec2 (θ) √ x2 −a2 x = asec(θ) 0, π 2 sec2 (θ) −1. Figure 7.3.7: calculating the area of the shaded region requires evaluating an integral with a trigonometric substitution. we can see that the area is a = ∫5 3√x2 − 9dx. to evaluate this definite integral, substitute x = 3secθ and dx = 3secθtanθdθ. we must also change the limits of integration. In general, when other methods fail, use the table below as a guide for certain types of integrals, making use of the specified substitution and trigonometric identity: for example, the substitution \(u = a\,\tan\,\theta\) leads to the following formula: similarly, the substitution \(u = a\,\sec\,\theta\) yields this formula:. With practice, you will gain insight into what kind of substitution will work best for a particular integral. key concepts trigonometric substitutions are often useful for integrals containing factors of the form \[(a^2 x^2)^n,\qquad\qquad (x^2 a^2)^n,\qquad {\small\textrm{or}}\qquad (x^2 a^2)^n.\].

The Six Basic Trigonometric Functions Shorts Trigonometry Trig Math In general, when other methods fail, use the table below as a guide for certain types of integrals, making use of the specified substitution and trigonometric identity: for example, the substitution \(u = a\,\tan\,\theta\) leads to the following formula: similarly, the substitution \(u = a\,\sec\,\theta\) yields this formula:. With practice, you will gain insight into what kind of substitution will work best for a particular integral. key concepts trigonometric substitutions are often useful for integrals containing factors of the form \[(a^2 x^2)^n,\qquad\qquad (x^2 a^2)^n,\qquad {\small\textrm{or}}\qquad (x^2 a^2)^n.\].

Basic Trigonometric Substitution Shorts Youtube