Basic Of Algebra Rules Operations And Formulas
Basic Of Algebra Rules Operations And Formulas An expression consisting of 4 main parts, variables, operators, exponents, coefficients and constants along with an equal to symbol is known as an algebraic equation. let us take an equation, ax 2 bx c = d. in algebra, the term with highest exponent is written in the starting and further the terms are written with reducing powers. Basic algebra operations. the general arithmetic operations performed in the case of algebra are: addition: x y. subtraction: x – y. multiplication: xy. division: x y or x ÷ y. where x and y are the variables. the order of these operations will follow the bodmas rule, which means the terms inside the brackets are considered first.

Basic Of Algebra Rules Operations And Formulas Unit 2: algebraic expressions. our mission is to provide a free, world class education to anyone, anywhere. khan academy is a 501 (c) (3) nonprofit organization. donate or volunteer today!. Substitute 9 into the problem, and you get 24 ÷ 3 11 – 9 × 2. then perform all multiplication and division. working left to right, 24 ÷ 3 = 8 and 9 × 2 = 18. substitute those numbers into the problem, and you have 8 11 – 18. finish with addition and subtraction. so, you end up with 8 11 – 18 = 19 – 18 = 1. Here are a few examples of equations using addition and subtraction. example 1. {eq}y 10 = 22 { eq} here we have subtraction happening on the left side. remember, we want to isolate the variable. Unit 2: solving basic equations & inequalities (one variable, linear) algebraic equations basics: solving basic equations & inequalities (one variable, linear) why we do the same thing to both sides of an equation: solving basic equations & inequalities (one variable, linear) one step addition & subtraction equations: solving basic equations.

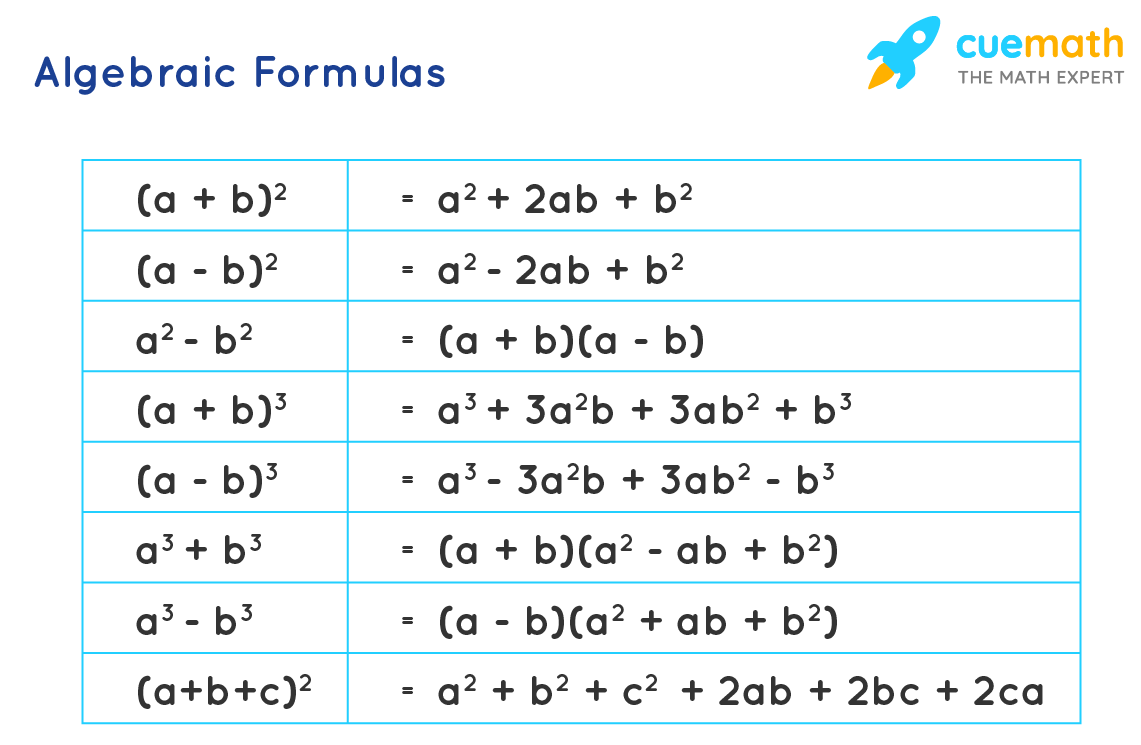
Algebra Basics Rules Operations Formula And Examples Here are a few examples of equations using addition and subtraction. example 1. {eq}y 10 = 22 { eq} here we have subtraction happening on the left side. remember, we want to isolate the variable. Unit 2: solving basic equations & inequalities (one variable, linear) algebraic equations basics: solving basic equations & inequalities (one variable, linear) why we do the same thing to both sides of an equation: solving basic equations & inequalities (one variable, linear) one step addition & subtraction equations: solving basic equations. Algebra is just like a puzzle where we start with something like "x − 2 = 4" and we want to end up with something like "x = 6". but instead of saying " obviously x=6", use this neat step by step approach: work out what to remove to get "x = " remove it by doing the opposite (adding is the opposite of subtracting) do that to both sides. Square of the sum: (a b)2 = a2 2ab b2. square of the difference: (a– b)2 = a2– 2ab b2. difference of squares: a2–b2 = (a b)(a– b) this quick example of the square of the sum formula, will help you see how this formula works in practice. the following formulas are useful when expanding and simplifying binomials.

Algebraic Rules Algebra is just like a puzzle where we start with something like "x − 2 = 4" and we want to end up with something like "x = 6". but instead of saying " obviously x=6", use this neat step by step approach: work out what to remove to get "x = " remove it by doing the opposite (adding is the opposite of subtracting) do that to both sides. Square of the sum: (a b)2 = a2 2ab b2. square of the difference: (a– b)2 = a2– 2ab b2. difference of squares: a2–b2 = (a b)(a– b) this quick example of the square of the sum formula, will help you see how this formula works in practice. the following formulas are useful when expanding and simplifying binomials.

Comments are closed.