Antiderivatives Section 5 7
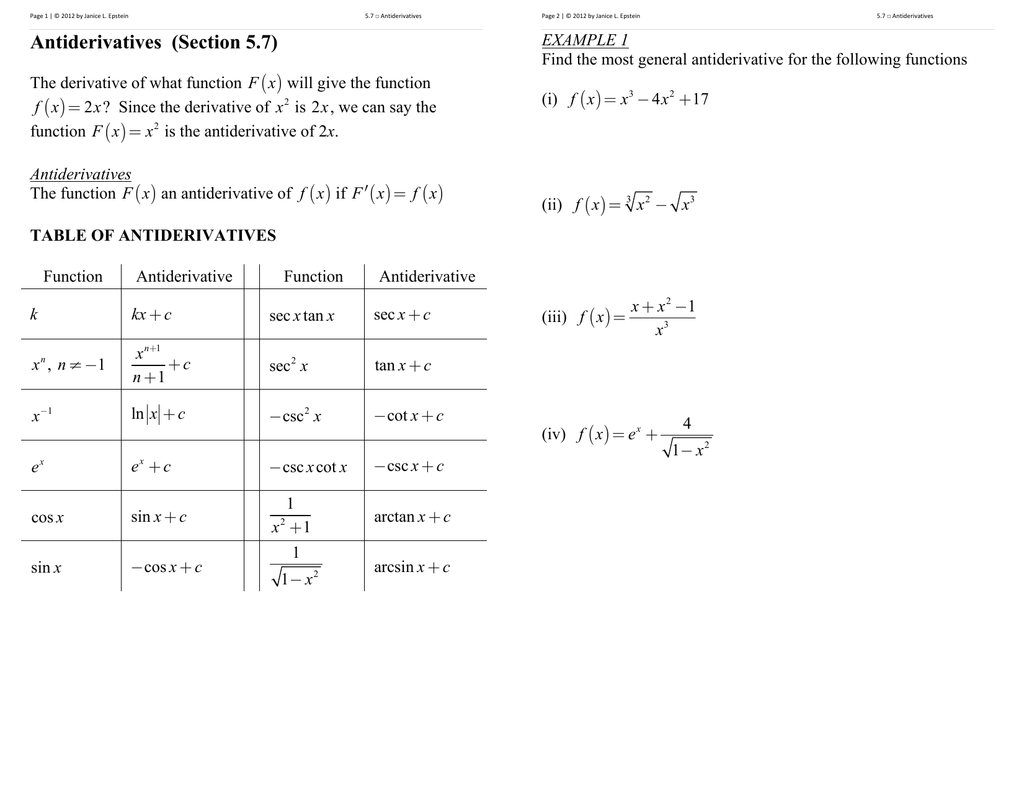
Antiderivatives Section 5 7 Section 5.7: antiderivatives definition 1. a function f is called an antiderivative of f on an interval i if f0(x) = f(x) for all x in i. example 2. (a) is the. In the following exercises, solve for the antiderivative ∫ f ∫ f of f with c = 0, c = 0, then use a calculator to graph f and the antiderivative over the given interval [a, b]. [a, b]. identify a value of c such that adding c to the antiderivative recovers the definite integral f (x) = ∫ a x f (t) d t. f (x) = ∫ a x f (t) d t.
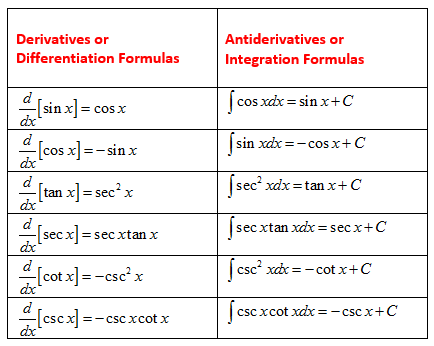
Calculus Antiderivative Video Lessons Examples Solutions In exercises 17 20, solve for the antiderivative of \(f\) with \(c=0\), then use a calculator to graph \(f\) and the antiderivative over the given interval \([a,b]\). identify a value of \(c\) such that adding \(c\) to the antiderivative recovers the definite integral \(\displaystyle f(x)=∫^x af(t)\,dt\) . Evaluate ∫ (4 x 3 − 5 x 2 x − 7) d x. ∫ (4 x 3 − 5 x 2 x − 7) d x. initial value problems we look at techniques for integrating a large variety of functions involving products, quotients, and compositions later in the text. And. d. arccos x dx. . x2. when listing the antiderivative that corresponds to each of the inverse trigonometric functions, you need to use only one member from each pair. it is conventional to use arcsin x as the antiderivative of 1 1 x2, rather than arccos x. the next theorem gives one antiderivative formula for each of the three pairs. the. Definition: indefinite integrals. given a function f, the indefinite integral of f, denoted. ∫f(x)dx, is the most general antiderivative of f. if f is an antiderivative of f, then. ∫f(x)dx = f(x) c. the expression f(x) is called the integrand and the variable x is the variable of integration. given the terminology introduced in this.

Antiderivatives And. d. arccos x dx. . x2. when listing the antiderivative that corresponds to each of the inverse trigonometric functions, you need to use only one member from each pair. it is conventional to use arcsin x as the antiderivative of 1 1 x2, rather than arccos x. the next theorem gives one antiderivative formula for each of the three pairs. the. Definition: indefinite integrals. given a function f, the indefinite integral of f, denoted. ∫f(x)dx, is the most general antiderivative of f. if f is an antiderivative of f, then. ∫f(x)dx = f(x) c. the expression f(x) is called the integrand and the variable x is the variable of integration. given the terminology introduced in this. Theorem 5.1.1: antiderivativeforms. let f(x) and g(x) be antiderivatives of f(x). then there exists a constant c such that. g(x) = f(x) c. given a function f and one of its antiderivatives f, we know all antiderivatives of f have the form f(x) c for some constant c. using definition 5.1.1, we can say that. Sections 5. 1 & 5.2:antiderivatives and inde nite integralsde nition. a function f is called an antiderivative of f. on an i. terval if f 0(x) = f(x) for all x in that interval.result. if f is an antiderivative. st general antiderivative ofon t. at interva.

Antiderivative Rules List Formulas Examples What Are Theorem 5.1.1: antiderivativeforms. let f(x) and g(x) be antiderivatives of f(x). then there exists a constant c such that. g(x) = f(x) c. given a function f and one of its antiderivatives f, we know all antiderivatives of f have the form f(x) c for some constant c. using definition 5.1.1, we can say that. Sections 5. 1 & 5.2:antiderivatives and inde nite integralsde nition. a function f is called an antiderivative of f. on an i. terval if f 0(x) = f(x) for all x in that interval.result. if f is an antiderivative. st general antiderivative ofon t. at interva.

A Comprehensive Guide To Antiderivatives And Integration In Course Hero

Comments are closed.