All About Parallel Lines Corresponding Angles Alternate Interior
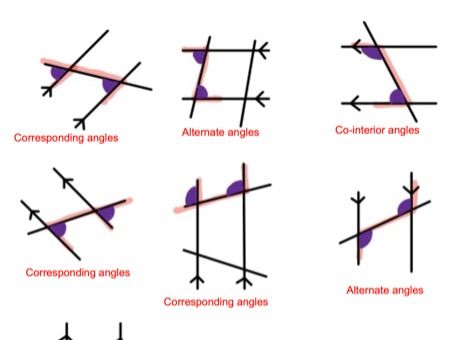
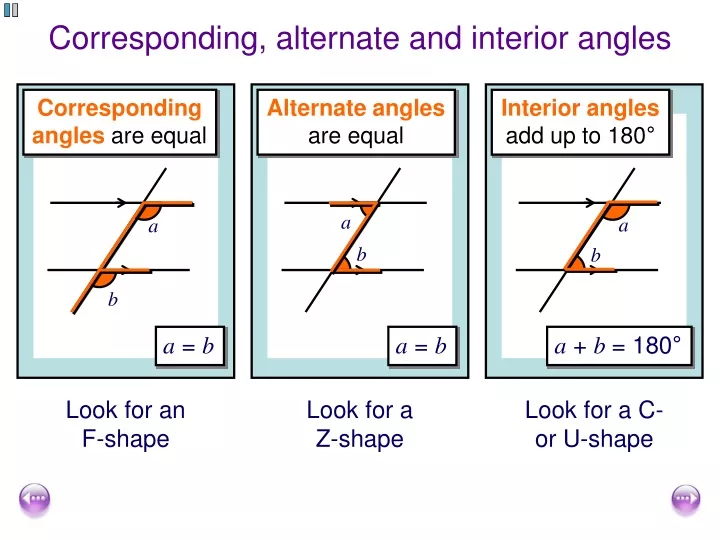
Angles Rules In Parallel Lines Alternate Co Interior Corresponding Types of angles. in geometry, there are many types of angles such as congruent, adjacent, vertical, corresponding, alternating, exterior, and interior angles. all angles have relationships to other angles and those angle relationships are what we will cover here. get free estimates from geometry tutors near you. Alternate interior angles. when two lines are crossed by another line (called the transversal): alternate interior angles are a pair of angles on the inner side of each of those two lines but on opposite sides of the transversal. in this example c and f are a pair of alternate interior angles. also d and e are a pair of alternate interior.
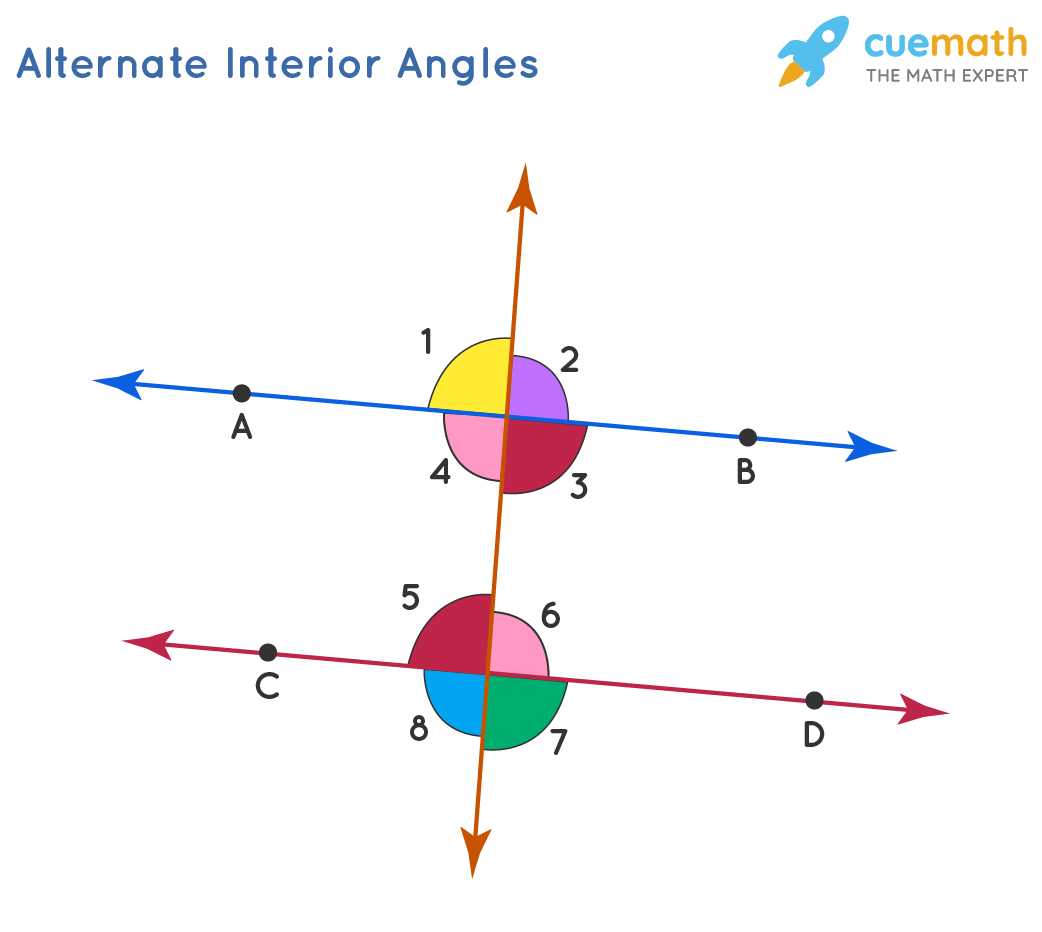
Alternate Interior Angles Definition Theorems Examples Alternate interior angles examples. example 1: find the measure of angle x in the following figure if the two lines are parallel and they are crossed by a transversal. solution: by the alternate interior angles theorem, x and 20° are the alternate interior angles. hence, they are equal. Pairs of angles. when a transversal cuts two parallel lines, a total of eight separate angles are formed – a through f in the figure below. from these eight angles, there are certain pairs of angles with special relationships connecting them – they are either equal or their sum is 180 ° \hspace{0.2em} 180 \degree \hspace{0.2em} 180°. These lines are parallel, because a pair of corresponding angles are equal. these lines are not parallel, because a pair of consecutive interior angles do not add up to 180° (81° 101° =182°) these lines are parallel, because a pair of alternate interior angles are equal. mathopolis: q1 q2 q3 q4 q5 q6 q7 q8 q9 q10. The angles which are formed inside the two parallel lines, when intersected by a transversal, are equal to its alternate pairs. these angles are called alternate interior angles. in the above given figure, you can see, two parallel lines are intersected by a transversal. therefore, the alternate angles inside the parallel lines will be equal.
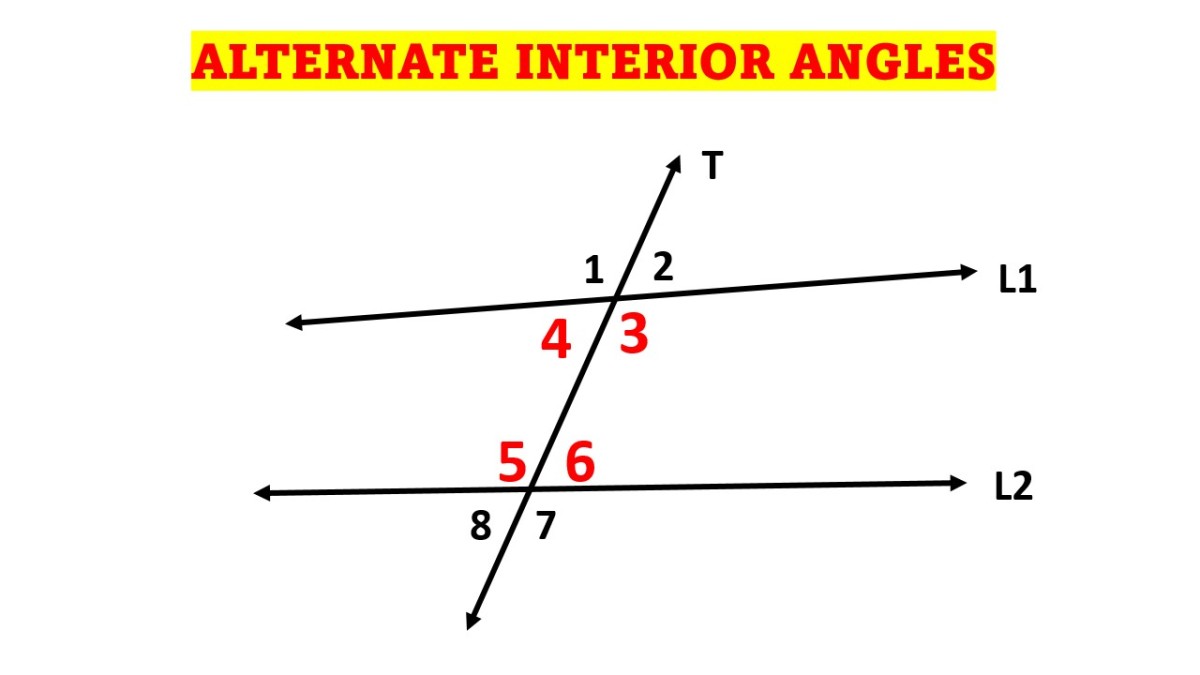
Alternate Interior Angles Examples Definition Theorem Education Tips These lines are parallel, because a pair of corresponding angles are equal. these lines are not parallel, because a pair of consecutive interior angles do not add up to 180° (81° 101° =182°) these lines are parallel, because a pair of alternate interior angles are equal. mathopolis: q1 q2 q3 q4 q5 q6 q7 q8 q9 q10. The angles which are formed inside the two parallel lines, when intersected by a transversal, are equal to its alternate pairs. these angles are called alternate interior angles. in the above given figure, you can see, two parallel lines are intersected by a transversal. therefore, the alternate angles inside the parallel lines will be equal. Alternate interior angles are formed when a transversal crosses two parallel or non parallel lines. one way to help you identify this angle pair is to look closely at the words alternate and interior. in our diagram above, both are located on the inner side or in between the lines . they are also on opposite sides of the transversal and are not. I) parallel lines cut by a transversal. ii) non parallel lines cut by a transversal. alternate interior angles are: ∠2 and ∠3. ∠1 and ∠4. if the lines are parallel, the alternate interior angles are always equal. in other words, the alternate interior angles can be used to prove whether the lines given are parallel or not.
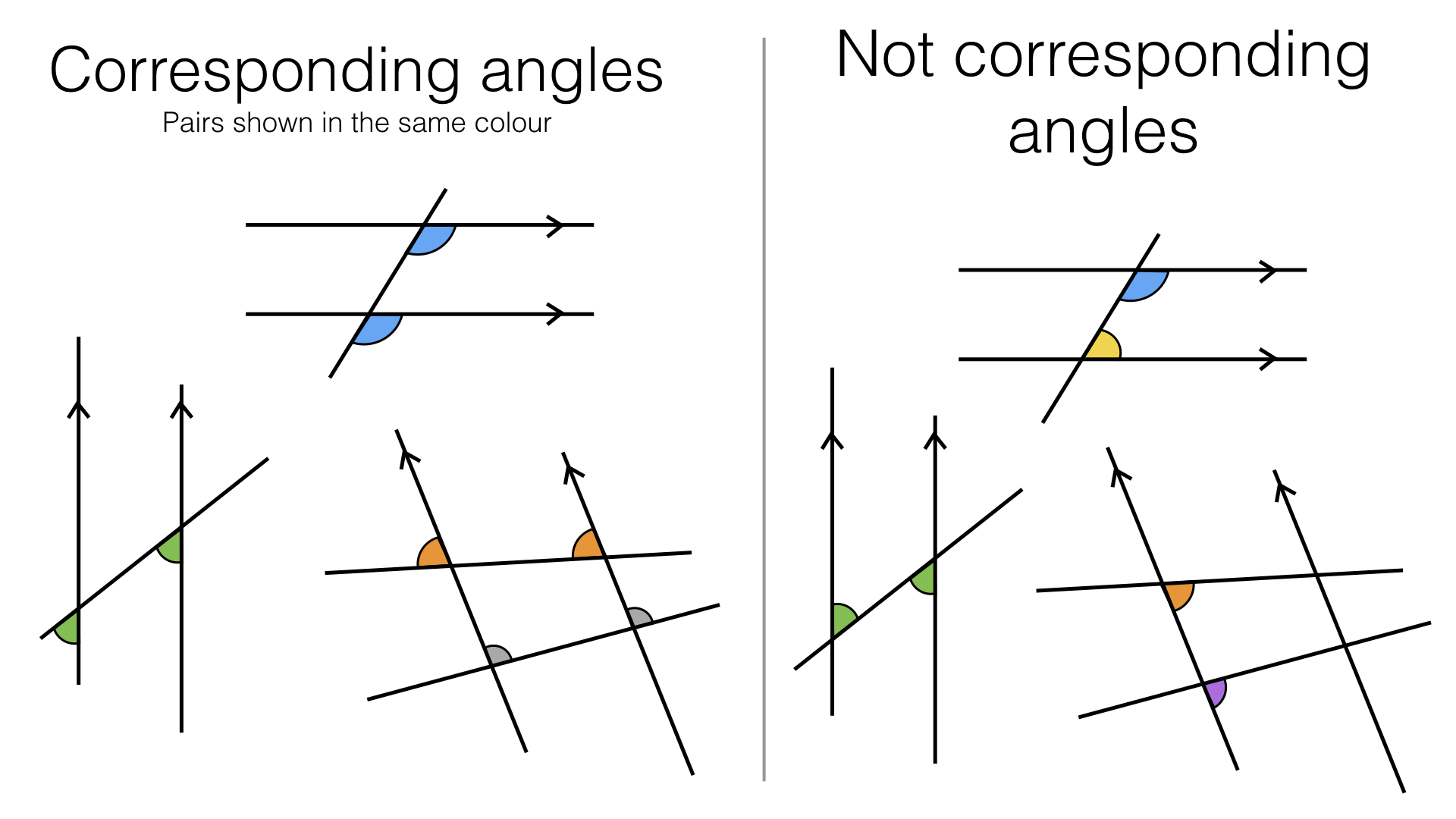
G3b Alternate And Corresponding Angles On Parallel Lines Bossmaths Alternate interior angles are formed when a transversal crosses two parallel or non parallel lines. one way to help you identify this angle pair is to look closely at the words alternate and interior. in our diagram above, both are located on the inner side or in between the lines . they are also on opposite sides of the transversal and are not. I) parallel lines cut by a transversal. ii) non parallel lines cut by a transversal. alternate interior angles are: ∠2 and ∠3. ∠1 and ∠4. if the lines are parallel, the alternate interior angles are always equal. in other words, the alternate interior angles can be used to prove whether the lines given are parallel or not.
Ppt Corresponding Alternate And Interior Angles Powerpoint

Alternate And Interior Angles In Parallel Lines Mr Mathematics

Comments are closed.