6 1 Angles Of Polygons
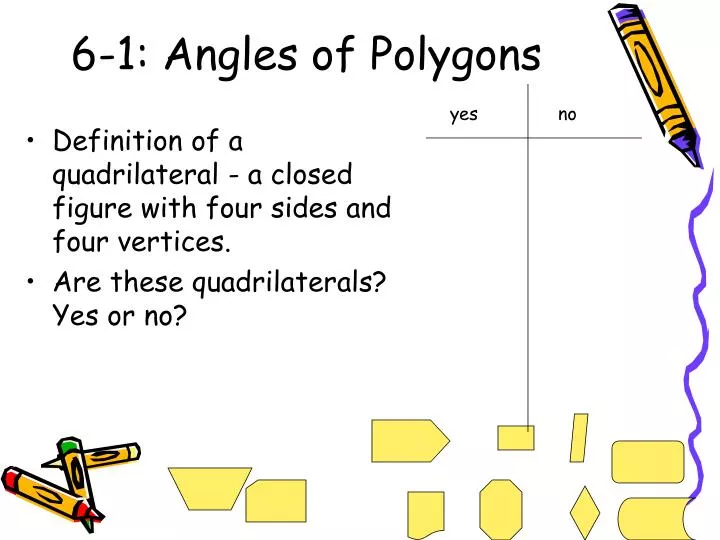
Ppt 6 1 Angles Of Polygons Powerpoint Presentation Free Download If it is a regular polygon (all sides are equal, all angles are equal) shape sides sum of interior angles shape each angle; triangle: 3: 180° 60° quadrilateral: 4: 360° 90° pentagon: 5: 540° 108° hexagon: 6: 720° 120° heptagon (or septagon) 7: 900° 128.57 ° octagon: 8: 1080° 135° nonagon: 9: 1260° 140° any polygon: n (n−2. Ck12.orgchapter 6. polygons and quadrilaterals exterior angle sum theorem: the sum of the exterior angles of any polygon is 360 . proof of the exterior angle sum theorem given: any n gon with n sides, n interior angles and n exterior angles. prove: n exterior angles add up to 360 note: the interior angles are x 1;x 2;:::x n. the exterior.
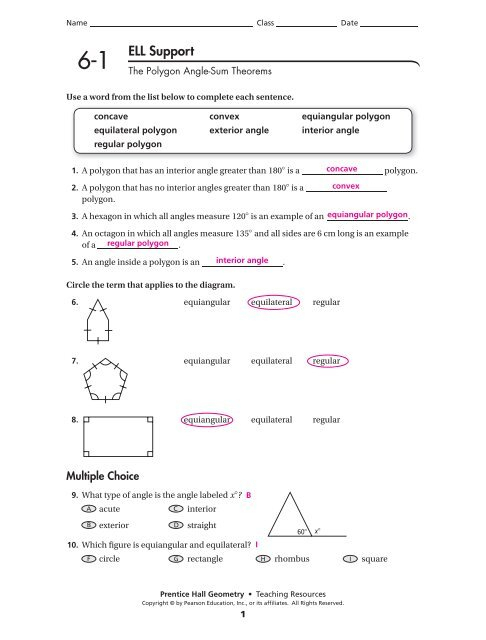
Geometry 6 1 Patterns Of Angles In Polygons Worksheet Answers The measure of an interior angle of a regular polygon is 150 degrees. how many sides must this polygon have? 24. if one exterior angle of a regular polygon measures 72°, what is the measure of one interior angle? 25. a portion of a regular polygon is shown above. How to determine the measures of the interior and exterior angles of a polygon and their sums. $$ \red 6 $$ sided polygon (hexagon) $$ (\red 6 2) \cdot 180 $$ $$ 720^{\circ} $$ problem 1 exterior angles of a polygon. formula for sum of exterior angles:. Scroll down the page for more examples and solutions on the interior angles of a polygon. example: find the sum of the interior angles of a heptagon (7 sided) solution: step 1: write down the formula (n 2) × 180°. step 2: plug in the values to get (7 2) × 180° = 5 × 180° = 900°. answer: the sum of the interior angles of a heptagon (7.

Section 6 1 Angles Of Polygons Ppt Download $$ \red 6 $$ sided polygon (hexagon) $$ (\red 6 2) \cdot 180 $$ $$ 720^{\circ} $$ problem 1 exterior angles of a polygon. formula for sum of exterior angles:. Scroll down the page for more examples and solutions on the interior angles of a polygon. example: find the sum of the interior angles of a heptagon (7 sided) solution: step 1: write down the formula (n 2) × 180°. step 2: plug in the values to get (7 2) × 180° = 5 × 180° = 900°. answer: the sum of the interior angles of a heptagon (7. 6.1 angles of. that connects outside of the polygon. polygon is when there is a diagonal. concave. connect inside the polygon. polygon is when all of the diagonals. convex. polygon names. sides congruent and all angles congruent. To find the sum of interior angles of a polygon, multiply the number of triangles in the polygon by 180°. the formula for calculating the sum of interior angles is \ ( (n 2) \times 180^\circ.
Ppt 6 1 Angles Of Polygons N Powerpoint 6.1 angles of. that connects outside of the polygon. polygon is when there is a diagonal. concave. connect inside the polygon. polygon is when all of the diagonals. convex. polygon names. sides congruent and all angles congruent. To find the sum of interior angles of a polygon, multiply the number of triangles in the polygon by 180°. the formula for calculating the sum of interior angles is \ ( (n 2) \times 180^\circ.

Comments are closed.