5 3 Multiplying Polynomials

Ppt Lesson 5 3 Multiplying Polynomials Powerpoint Presentation Free Ai explanations are generated using openai technology. ai generated content may present inaccurate or offensive content that does not represent symbolab's view. middle school math solutions – polynomials calculator, multiplying polynomials. multiplying polynomials can be tricky because you have to pay attention to every term, not to mention. Multiply: ⓐ 4 x 2 (2 x 2 − 3 x 5) 4 x 2 (2 x 2 − 3 x 5) ⓑ −6 a 3 b (3 a 2 − 2 a b 6 b 2). −6 a 3 b (3 a 2 − 2 a b 6 b 2). multiply a binomial by a binomial just like there are different ways to represent multiplication of numbers, there are several methods that can be used to multiply a binomial times a binomial.

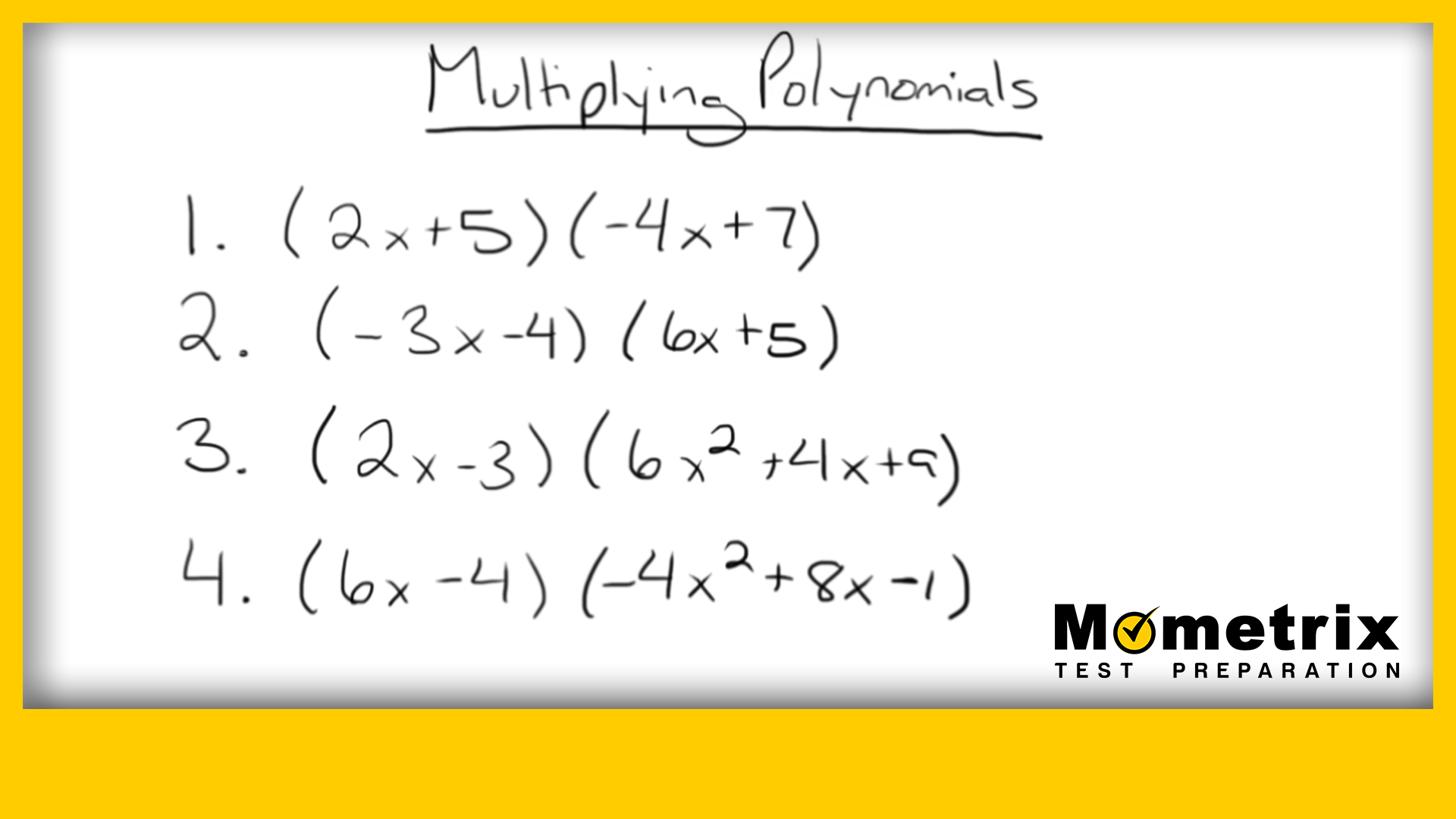
5 3 Multiplying Polynomials 1 Youtube Multiplying polynomials. a polynomial looks like this: example of a polynomial. this one has 3 terms. to multiply two polynomials: multiply each term in one polynomial by each term in the other polynomial. add those answers together, and simplify if needed. let us look at the simplest cases first. Example: multiply 5x 2 with 3y. step 1: we will first multiply the coefficients of both the polynomials i.e., 5 × 3= 15. step 2: since the above polynomials have two different variables, they cannot be multiplied. hence, we will keep them the same. the final answer is 5x 2 × 3y = 15x 2 y. Answer: to summarize, multiplying a polynomial by a monomial involves the distributive property and the product rule for exponents. multiply all of the terms of the polynomial by the monomial. for each term, multiply the coefficients and add exponents of variables where the bases are the same. exercise 5.4.1. This is where using the distributive property (or distributive method) will help you! to multiply these polynomials, start by taking the first polynomial (the purple monomial) and multiplying it by each term in the second polynomial (the green trinomial). this can be done by multiplying 4x^2 by the first term of the green trinomial (figure 1.
Multiplying Polynomials Practice Video Answer: to summarize, multiplying a polynomial by a monomial involves the distributive property and the product rule for exponents. multiply all of the terms of the polynomial by the monomial. for each term, multiply the coefficients and add exponents of variables where the bases are the same. exercise 5.4.1. This is where using the distributive property (or distributive method) will help you! to multiply these polynomials, start by taking the first polynomial (the purple monomial) and multiplying it by each term in the second polynomial (the green trinomial). this can be done by multiplying 4x^2 by the first term of the green trinomial (figure 1. Write the first binomial, x – 4 –4 vertically and the second binomial, x 2, horizontally. then multiply the corresponding terms and place the partial products in their individual square grid. now, add up all the terms inside the square grids. make sure to combine like terms which in this case the terms. finish this off by combining like. Multiply: (y2 7)(y − 9) (2xy 3)(4xy − 5). answer. the foil method is usually the quickest method for multiplying two binomials, but it only works for binomials. you can use the distributive property to find the product of any two polynomials. another method that works for all polynomials is the vertical method.

5 3 Multiplying Polynomials Youtube Write the first binomial, x – 4 –4 vertically and the second binomial, x 2, horizontally. then multiply the corresponding terms and place the partial products in their individual square grid. now, add up all the terms inside the square grids. make sure to combine like terms which in this case the terms. finish this off by combining like. Multiply: (y2 7)(y − 9) (2xy 3)(4xy − 5). answer. the foil method is usually the quickest method for multiplying two binomials, but it only works for binomials. you can use the distributive property to find the product of any two polynomials. another method that works for all polynomials is the vertical method.

Comments are closed.