14 Laws Of Logarithms Ppt Logarithm Algebra

14 Laws Of Logarithms Ppt Logarithm Algebra 14 laws of logarithms.ppt free download as powerpoint presentation (.ppt), pdf file (.pdf), text file (.txt) or view presentation slides online. the document discusses three logarithm laws: the product rule, quotient rule, and power rule. it provides examples of applying each rule to evaluate logarithmic expressions. The document discusses logarithms and includes examples of solving logarithmic equations, graphing logarithmic functions, and properties of logarithms and the natural logarithm function. key points covered include: logarithms are used to solve for exponents. common properties include loga1=0, logaa=1, and logaax=x.

Rules Of Logarithms With Examples Intro to logarithms.ppt google slides. 1 introduction to logarithms 2 logarithms were originally developed to simplify complex arithmetic calculations. they were designed to transform multiplicative processes into additive ones. 3 if at first this seems like no big deal, then try multiplying 2,234,459,912 and 3,456,234,459. without a calculator. The logarithm of the ratio of two quantities is the logarithm of the numerator minus the logarithm of the denominator. the logarithm of an exponential number is the exponent times the logarithm of the base. the logarithm of the argument (inside the parenthesis) wherein the argument equals the base is equal to . for for b> 0 b>0. Logarithms were invented to solve exponential equations like 2x = 6, where x is between 2 and 3. a logarithm with base b is defined as logby = x if and only if bx = y. common properties of logarithms include logb1 = 0 and logbb = 1. logarithmic functions have inverse relationships with exponential functions and can be graphed by shifting and. The document summarizes several laws of exponents and logarithms: the product rule for exponents states that xm × xn = xm n, and the quotient rule states that xm xn = xm n. similarly, the product rule for logarithms is logb(xy) = logb(x) logb(y), and the quotient rule is logb(x y) = logb(x) logb(y). examples are given to illustrate how to use these rules to simplify logarithmic.

Law Of Logarithms With Examples Logarithms were invented to solve exponential equations like 2x = 6, where x is between 2 and 3. a logarithm with base b is defined as logby = x if and only if bx = y. common properties of logarithms include logb1 = 0 and logbb = 1. logarithmic functions have inverse relationships with exponential functions and can be graphed by shifting and. The document summarizes several laws of exponents and logarithms: the product rule for exponents states that xm × xn = xm n, and the quotient rule states that xm xn = xm n. similarly, the product rule for logarithms is logb(xy) = logb(x) logb(y), and the quotient rule is logb(x y) = logb(x) logb(y). examples are given to illustrate how to use these rules to simplify logarithmic. This law tells us how to add two logarithms together. adding log a and log b results in the logarithm of the product of a and b, that is log ab. for example, we can write. log10 5 log10 4 = log10(5 × 4) = log10 20. the same base, in this case 10, is used throughout the calculation. you should verify this by evaluating both sides separately. Presentation transcript: 1 4.4 laws of logarithms. 2 laws of logarithms since logarithms are exponents, the laws of exponents give rise to the laws of logarithms. 3 example 1 – using the laws of logarithms to evaluate expressions. evaluate each expression. (a) log4 2 log4 32 (b) log2 80 – log2 5 (c) log 8 solution: (a) log4 2 log4 32.

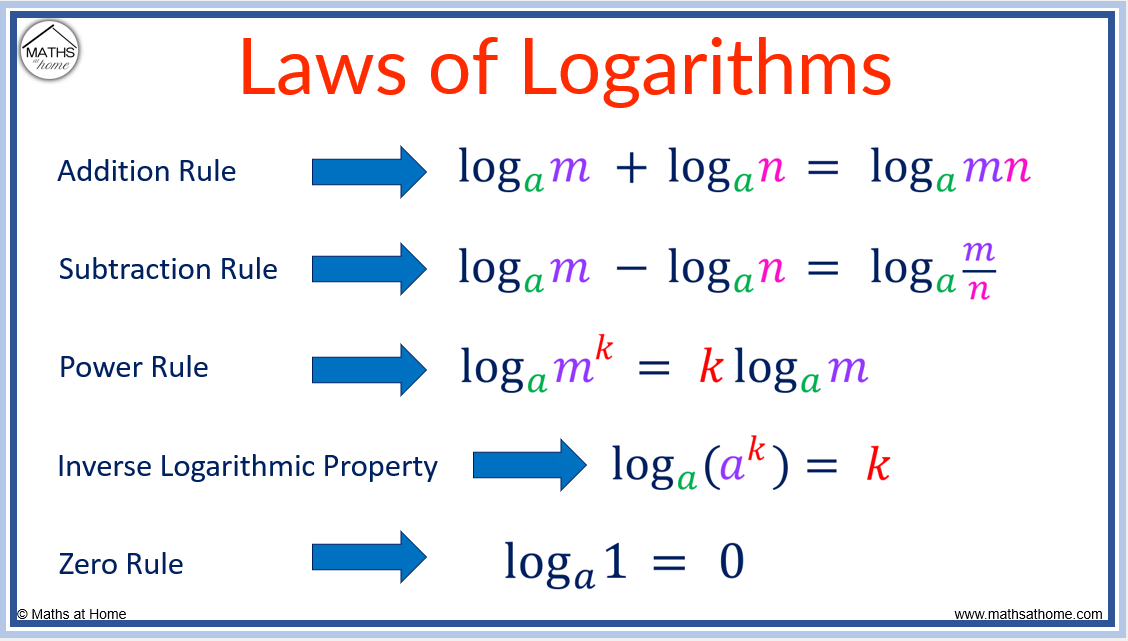
Logarithm Laws Made Easy A Complete Guide With Examples Mathsathome This law tells us how to add two logarithms together. adding log a and log b results in the logarithm of the product of a and b, that is log ab. for example, we can write. log10 5 log10 4 = log10(5 × 4) = log10 20. the same base, in this case 10, is used throughout the calculation. you should verify this by evaluating both sides separately. Presentation transcript: 1 4.4 laws of logarithms. 2 laws of logarithms since logarithms are exponents, the laws of exponents give rise to the laws of logarithms. 3 example 1 – using the laws of logarithms to evaluate expressions. evaluate each expression. (a) log4 2 log4 32 (b) log2 80 – log2 5 (c) log 8 solution: (a) log4 2 log4 32.