1 1 2 1 2 3 1 Nn 1 N N 1 Prove The Given Statement By Induction For A
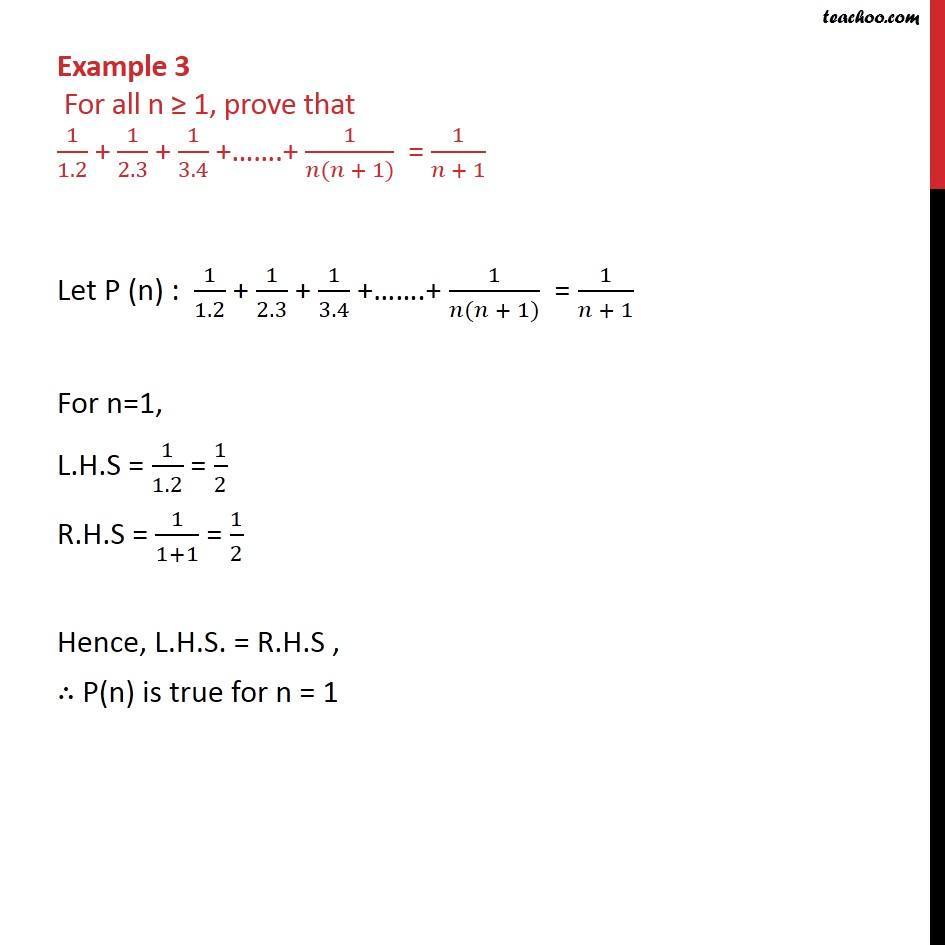
Example 3 Prove 1 1 2 1 2 3 1 3 4 1 N N 1 1 N 1 Induction, the given statement is true for every positive integer n. 3. 13 23 33 n3 = n2(n 1)2 4 proof: for n = 1, the statement reduces to 13 = 12 22 4 and is obviously true. assuming the statement is true for n = k: 13 23 33 k3 = k2(k 1)2 4; (5) we will prove that the statement must be true for n = k 1: 13 23 33. Stack exchange network. stack exchange network consists of 183 q&a communities including stack overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.

Prove 1 2 3 N N N 1 2 Mathematical Induction Answer: 1 2 3 2 5 2 (2n 1) 2 = n(2n 1)(2n 1) 3 is true for all positive integers n. example 2: prove that 2 n > n for all positive integers n. solution: we will prove the result using the principle of mathematical induction. Process of proof by induction. there are two types of induction: regular and strong. the steps start the same but vary at the end. here are the steps. in mathematics, we start with a statement of our assumptions and intent: let p(n), ∀n ≥ n0, n, n0 ∈ z be a statement. we would show that p (n) is true for all possible values of n. To prove the implication. p(k) ⇒ p(k 1) (3.6.3) (3.6.3) p (k) ⇒ p (k 1) in the inductive step, we need to carry out two steps: assuming that p(k) p (k) is true, then using it to prove p(k 1) p (k 1) is also true. so we can refine an induction proof into a 3 step procedure: verify that p(a) p (a) is true. Axiom, we therefore have that s = n, so every natural number n satis es the equation 1 2 3 n = n(n 1) 2: we can simplify this technique to apply to the general statement in (1). let’s think carefully about what we really needed to prove in the above example. i will refer to the statement 1 2 n = n(n 1) 2 as p(n) here. we speci.

Prove 1 2 3 N N N 1 2 Mathematical Induction To prove the implication. p(k) ⇒ p(k 1) (3.6.3) (3.6.3) p (k) ⇒ p (k 1) in the inductive step, we need to carry out two steps: assuming that p(k) p (k) is true, then using it to prove p(k 1) p (k 1) is also true. so we can refine an induction proof into a 3 step procedure: verify that p(a) p (a) is true. Axiom, we therefore have that s = n, so every natural number n satis es the equation 1 2 3 n = n(n 1) 2: we can simplify this technique to apply to the general statement in (1). let’s think carefully about what we really needed to prove in the above example. i will refer to the statement 1 2 n = n(n 1) 2 as p(n) here. we speci. Proof by induction. step 1: prove the base case. this is the part where you prove that \ (p (k)\) is true if \ (k\) is the starting value of your statement. the base case is usually showing that our statement is true when \ (n=k\). step 2: the inductive step. Theorem 1.3.1: principle of mathematical induction. for each natural number n ∈ n, suppose that p(n) denotes a proposition which is either true or false. let a = {n ∈ n: p(n) is true }. suppose the following conditions hold: 1 ∈ a. for each k ∈ n, if k ∈ a, then k 1 ∈ a. then a = n.

Prove That 1 3 2 3 3 3 N 3 N N 1 2 2 Teachoo Proof by induction. step 1: prove the base case. this is the part where you prove that \ (p (k)\) is true if \ (k\) is the starting value of your statement. the base case is usually showing that our statement is true when \ (n=k\). step 2: the inductive step. Theorem 1.3.1: principle of mathematical induction. for each natural number n ∈ n, suppose that p(n) denotes a proposition which is either true or false. let a = {n ∈ n: p(n) is true }. suppose the following conditions hold: 1 ∈ a. for each k ∈ n, if k ∈ a, then k 1 ∈ a. then a = n.

Question 14 Prove 1 1 1 1 1 2 1 1 3 1 1 N

Comments are closed.