03 Negative Exponents Powers Of Zero Laws Of Exponents Part 1

03 Negative Exponents Powers Of Zero Laws Of Exponents Part 1 View more at mathtutordvd .in this lesson, you will learn how to simplify expressions that involve negative exponents in algebra. first, we wi. According to the exponent rules, to multiply two expressions with the same base, we add the exponents while the base remains the same. this means, 10 3 × 10 4 = 10 ( 3 4) = 10 1 = 10. answer: 10. example 2: simplify the given expression and select the correct option using the laws of exponents: 10 15 ÷ 10 7. (a) 10 8.

03 Negative Exponents Powers Of Zero Laws Of Exponents Part 1 The exponent says how many times to use the number in a multiplication. a negative exponent means divide, because the opposite of multiplying is dividing. a fractional exponent like 1 n means to take the nth root: x (1 n) = n√x. if you understand those, then you understand exponents!. Rule 2. 1 a − n = an. rule 3. (a b) − n = (b a)n. negative exponents are combined in several different ways. as a general rule, in a fraction, a base with a negative exponent moves to the other side of the fraction bar as the exponent changes sign. Now, let’s go over the seven (7) basic exponent rules or laws. any nonzero number raised to zero power is equal to 1. we have a nonzero base of , and an exponent of zero. the zero rule of exponent can be directly applied here. thus, {5^0} = 1. the base here is the entire expression inside the parenthesis, and the good thing is that it is. A negative exponent means how many times to divide by the number. example: 8 1 = 1 ÷ 8 = 1 8 = 0.125. or many divides: example: 5 3 = 1 ÷ 5 ÷ 5 ÷ 5 = 0.008. but that can be done an easier way: 5 3 could also be calculated like: 1 ÷ (5 × 5 × 5) = 1 53 = 1 125 = 0.008. that last example showed an easier way to handle negative exponents: so.

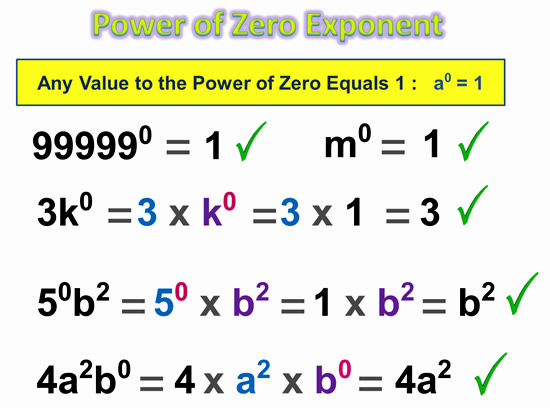
Zero And Negative Exponents Passy S World Of Mathematics Now, let’s go over the seven (7) basic exponent rules or laws. any nonzero number raised to zero power is equal to 1. we have a nonzero base of , and an exponent of zero. the zero rule of exponent can be directly applied here. thus, {5^0} = 1. the base here is the entire expression inside the parenthesis, and the good thing is that it is. A negative exponent means how many times to divide by the number. example: 8 1 = 1 ÷ 8 = 1 8 = 0.125. or many divides: example: 5 3 = 1 ÷ 5 ÷ 5 ÷ 5 = 0.008. but that can be done an easier way: 5 3 could also be calculated like: 1 ÷ (5 × 5 × 5) = 1 53 = 1 125 = 0.008. that last example showed an easier way to handle negative exponents: so. Return to the quotient rule. we made the condition that [latex]m>n[ latex] so that the difference [latex]m n[ latex] would never be zero or negative. what would happen if [latex]m=n[ latex]? in this case, we would use the zero exponent rule of exponents to simplify the expression to 1. to see how this is done, let us begin with an example. Exponents 0 and 1these laws were discussed in the video called “expone. ts in algebra”. the first law tell you that anything raised to the 0th. ower is always 1. the second law tells you that anything raised to the 1st pow. egative exponentsthis important law helps us understand that negative exponents are essentially.